In simple terms, the stochastic frontier approach amounts to specifying the relationship between output and input levels and using two error terms. One error term is the traditional normal error term in which the mean is zero and the variance is constant. The other error term represents technical inefficiency and may be expressed as a half-normal, truncated normal, exponential, or two-parameter gamma distribution. Technical efficiency is subsequently estimated via maximum likelihood of the production function subject to the two error terms.
Letting U be the technical inefficiency error term, technical efficiency is estimated as the ratio of the expected value of the predicted frontier output conditional on the value of U to the expected value of the predicted frontier output conditional on the value of U being 0.0:
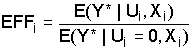
where E is the expectations operator, Y* is the predicted frontier output, U is the error term for technical inefficiency, and X is the vector of inputs used to produce Y. Capacity output is subsequently estimated to equal the frontier output for which U equals 0.0. A primal-based measure of capacity utilization may then be determined by calculating the ratio of observed output to the frontier output; this can be done for the individual firm or for the industry. The industry capacity output simple equals the sum of the frontier outputs; the industry capacity utilization equals the sum of observed outputs divided by the industry capacity output. Greene (1993) provides an extensive survey of stochastic production frontiers.
The stochastic frontier typically permits assessment of maximal output subject to input levels; as such, it appears to be an output-oriented measure. The stochastic frontier is, in fact, a base or nonorienting measure. That is, the assessment of efficiency is not conditional on holding all inputs or all outputs constant. Utilizing the one-stage routine of Battese and Coelli (1993), however, facilitates an assessment of maximal output from an input-based perspective. With this approach, the inefficiency error term, and subsequently the maximal output, is specified as a function of inputs. Thus, it is possible to consider the input reduction coinciding with a fixed maximum or frontier output.
A major criticism of the frontier approach is that it can not adequately handle multiple outputs. Two frameworks have been developed in the literature to overcome the criticisms related to multiple product technology: (1) the stochastic distance function, and (2) polar coordinates. Fare et al. (1993) introduce the concept of using distance functions to express the output bundle of a multiple product technology. Subsequently, the distance function is specified as a function of variable and fixed inputs and output levels. The technology is specified as a translog function and subsequently estimated by linear programming procedures. Coelli and Perelman (1996a, 1996b) provide state-of-the-art discussions of econometric estimation of stochastic distance functions with multiple outputs. Multiproduct stochastic distance functions suffer from input-output separability and linear homogeneity in outputs.
The polar coordinate framework as illustrated by Lothgren (1997) is similar. Lothgren specifies a translog flexible function form of a multiple product technology. The dependent variable is specified as distance function relative to the distances of all outputs from the origin. The independent variables are the usual factors of production but include polar coordinate values obtained relative to the various outputs. Estimation is accomplished by conventional maximum likelihood procedures with two error terms, as in the single product stochastic frontier approach. Although the procedures of Lothgren offer a possible way for estimating the stochastic frontier of multiple product technologies, there is likely a problem of implicit simultaneous equation bias in that functions of the dependent variable appear on both sides of the equation.