J. Kövári
Food and Agriculture Organization of
the United Nations
Rome, Italy
1. LIST OF SYMBOLS, DIMENSIONS AND UNITS
2. DESIGN FORMULAS FOR CHANNEL FLOW
3. DESIGN FORMULAS FOR HYDRAULIC STRUCTURES
4. DISCHARGE OF WELLS
5. DESIGN FORMULA FOR SCREEN
6. DESIGN FORMULA FOR FILTER
7. DESIGN FORMULAS FOR FLOW IN PIPES
8. DESIGN FORMULAS FOR PUMPING
A list of symbols with their dimensions and units used in the formulas is given in Table 1.
Table 1. Symbols, Dimensions and Units used
|
Symbol |
Description |
Dimensions |
Units |
|
A |
Area |
L2 |
m2 |
|
B |
Surface width of a channel |
L |
m |
|
b |
Bottom width of a channel |
L |
m |
|
D |
Diameter |
L |
m |
|
d |
depth |
L |
m |
|
g |
Acceleration due to the force of gravity |
L/T2 |
m/sec2 |
|
H |
Total head; head over a crest |
L |
m |
|
h |
Head or water depth |
L |
m |
|
hl |
Head loss |
L |
m |
|
k |
Permeability coefficient |
L/T |
m/sec |
|
L |
Length |
L |
m |
|
l |
Length |
L |
m |
|
n |
Manning's roughness coefficient |
T/L1/3 |
sec/m1/3 |
|
Pw |
Wetted perimeter |
L |
m |
|
|
Allowable pressure head for siphon |
L |
m |
|
|
Pressure |
L |
m |
|
Q |
Discharge |
L3/T |
m3/sec |
|
q |
Unit discharge |
L3/T |
m3/sec |
|
R |
Hydraulic radius |
L |
m |
|
r |
Radius |
L |
m |
|
s |
Drawdown |
L |
m |
|
V |
Volume |
L3 |
m3 |
|
v |
Average velocity |
L/T |
m/sec |
|
W |
Weight |
F |
kg |
|
w |
Unit weight of water; width of a structure |
F/L3; L |
kg/m3; m |
|
g |
Density of water |
F/L3 |
kg/m3 |
Symbols for Dimensionless Quantities
|
Symbol |
Quantity |
|
C |
Discharge coefficient |
|
Ks |
Screen loss coefficient |
|
S |
Bottom slope |
|
z |
Ratio of the side slope for a channel cross-section (horizontal to vertical) |
|
h |
Efficiency |
|
l |
Friction factor |
|
p |
3.1416 |
|
f |
Velocity coefficient |
|
y |
Contraction coefficient |
Manning's formula (Chow, 1959)
|
|
(2.1) |
where
v = average velocity, m/sec
R == hydraulic radius, m
A = cross-sectional area of the channel, m2
Pw = wetted perimeter of the channel, m
S = slope of the channel
n = roughness coefficient
The values of n for various channel conditions are illustrated in Table 2.
Discharge formula
|
|
m3/sec |
(2.2) |
Normal water depth formula
|
|
, m |
(2.3) |
Slope formula
|
|
(2.4) |
where
b = bottom width of the channel, m
z = ratio of the side slope
Table 2 Values of the Roughness Coefficient n (Simon, 1976)
|
Channel condition |
Value of n |
|
Exceptionally smooth, straight surfaces: enamelled or glazed coating; glass; lucite; brass |
0.009 |
|
Very well planed and fitted boards; smooth metal; pure cement plaster; smooth tar or paint coating |
0.010 |
|
Planed lumber; smoothed mortar (1/3 sand) without projections, in straight alignment |
0-011 |
|
Carefully fitted but unplaned boards, steel trowelled concrete in straight alignment |
0.012 |
|
Reasonably straight, clean, smooth surfaces without projections; good boards; carefully built brick wall; wood trowelled concrete; smooth, dressed ashlar |
0.013 |
|
Good wood, metal, or concrete surfaces with some curvature, very small projections, slight moss or algae growth or gravel deposition. Shot concrete surfaced with trowelled mortar |
0.014 |
|
Rough brick; medium quality cut stone surface; wood with algae or moss growth; rough concrete; riveted steel |
0.015 |
|
Very smooth and straight earth channels, free from growth; stone rubble set in cement; shot, untrowelled concrete deteriorated brick wall; exceptionally well excavated and surfaced channel cut in natural rock |
0.017 |
|
Well-built earth channels covered with thick, uniform silt deposits; metal flumes with excessive curvature, large projections, accumulated debris |
0.018 |
|
Smooth, well-packed earth; rough stone walls; channels excavated in solid, soft rock; little curving channels in solid loess, gravel or clay, with silt deposits, free from growth, in average condition; deteriorating uneven metal flume with curvatures and debris; very large canals in good condition |
0.020 |
|
Small, manmade earth channels in well-kept condition; straight natural streams with rather clean, uniform bottom without pools and flow barriers, cavings and scours of the banks |
0.025 |
|
Ditches; below average manmade channels with scattered cobbles in bed |
0.028 |
|
Well-maintained large floodway; unkempt artificial channels with scours, slides, considerable aquatic growth; natural stream with good alignment and fairly constant cross-section |
0.030 |
|
Permanent alluvial rivers with moderate changes in cross-section, average stage; slightly curving intermittent streams in very good condition |
0.033 |
|
Small, deteriorated artificial channels, half choked with aquatic growth, winding river with clean bed, but with pools and shallows |
0.035 |
|
Irregularly curving permanent alluvial stream with smooth bed; straight natural channels with uneven bottom, sand bars, dunes, few rocks and underwater ditches; lower section of mountainous streams with well-developed channel with sediment deposits; intermittent streams in good condition; rather deteriorated artificial channels, with moss and reeds, rocks, scours and slides |
0.040 |
|
Artificial earth channels partially obstructed with debris, roots, and weeds; irregularly meandering rivers with partly grown-in or rocky bed; developed flood plains with high grass and bushes |
0.067 |
|
Mountain ravines; fully ingrown small artificial channels; flat flood plains crossed by deep ditches (slow flow) |
0.080 |
|
Mountain creeks with waterfalls and steep ravines; very irregular flood plains; weedy and sluggish natural channels obstructed with trees |
0-10 |
|
Very rough mountain creeks, swampy, heavily vegetated rivers with logs and driftwood on the bottom; flood plain forest with pools |
0.133 |
|
Mudflows; very dense flood plain forests; watershed slopes |
0.22 |
Table 3 Allowable Mean Velocities against Erosion or Scour in Channels of various Soils and Materials
|
Description |
v, m/sec |
|
Soft clay or very fine clay |
0-2 |
|
Very fine or very light pure sand |
0.3 |
|
Very light loose sand or silt |
0.4 |
|
Coarse sand or light sandy soil |
0.5 |
|
Average sandy soil and good loam |
0.7 |
|
Sandy loam |
0.8 |
|
Average loam or alluvial soil |
0.9 |
|
Firm loam, clay loam |
1.0 |
|
Firm gravel or clay |
1.1 |
|
Stiff clay soil; ordinary gravel soil, or clay and gravel |
1.4 |
|
Broken stone and clay |
1.5 |
|
Grass |
1.2 |
|
Coarse gravel, cobbles, shale |
1.8 |
|
Conglomerates, cemented gravel, soft slate, tough hardpan, soft sedimentary rock |
1.8 - 2.5 |
|
Soft rock |
1.4 - 2.5 |
|
Hard rock |
3.0 - 4.6 |
|
Very hard rock or cement concrete (1:2:4 minimum) |
4.6-7.6 |
Table 4 Allowable Side Slopes for Trapezoidal Channels in various Soils (Davis, 1952)
|
Type of soil |
z |
|
Light sand, wet clay |
3:1 |
|
Wet sand |
2.5:1 |
|
Loose earth, loose sandy loam |
2:1 |
|
Ordinary earth, soft clay, sandy loam, gravelly loam or loam |
1.5:1 |
|
Ordinary gravel |
1.25:1 |
|
Stiff earth or clay, soft moorum |
1:1 |
|
Tough hard pan, alluvial soil, firm gravel, hard compact earth, hard moorum |
0.5:1 |
|
Soft rock |
0.25:1 |
Table 5 Characteristic Dimensions of Optimum Trapezoidal Channel for given Gross-sectional Area and Side Slope
|
Side slope |
|
|
|
|
|
|
0.5:1 |
0.759 |
0.938 |
1.698 |
2.640 |
0.379 |
|
1:1 |
0.739 |
0.612 |
2.092 |
2.705 |
0.370 |
|
1.5:1 |
0.689 |
0.417 |
2.483 |
2.905 |
0.344 |
|
2:1 |
0.636 |
0.300 |
2.844 |
3.145 |
0.318 |
|
2.5:1 |
0.589 |
0.227 |
3.169 |
3.395 |
0.295 |
|
3:1 |
0.549 |
0.174 |
3502 |
3.645 |
0.275 |
where:
h = water depth, m
b = bottom width, m
B = surface width, m
P = wetted perimeter, m
R = hydraulic radius, m
A = cross-sectional area, m2
Example 1
A trapezoidal earth channel of 1.5:1 side slopes is to be built on a slope of S = 0.001 to carry Q = 1.0 m3/sec. Design the channel cross-section such that the hydraulic radius is optimal.
Solution;
Using Figure 1 first we mark off the length of the 1.0 m3 /sec discharge on the edge of a sheet of paper. Next, keeping the line horizontal we place the paper's edge on the upper graphs, moving it upward along the corresponding slope S = 0.001 and shape (1.5:1) lines. Where the distance between the lines equals the discharge length we note the magnitude of the hydraulic radius R.
R = 0.40 m
The corresponding velocity in the channel is
v = 0.70 m/sec
obtained from the slope graph. The cross-sectional area is:
A = 1.43 m2
from the shape graph.
Entering the left bottom graph along the R = 0.40 curve, we find the intercept with the radial line indicating optimum conditions. In this case for
R = Ropt = 0.40 m
we get
b = 0.50 m
and
h = 0.82 m
Example 2
Design a channel in firm loam, for a discharge of 1 500 l/sec, at maximum permissible velocity.
Solution
From Table 3, the maximum allowable velocity in firm loam is v = 1,0 m/sec. From Table 4 assume side slopes of 1.5:1. From Table 2 the roughness coefficient is defined as n = 0.025.
Canal properties
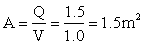
From Table 5
|
|
|
|
|
|
|
|
|
|
|
|
Figure 1. Lenkei's channel design graphs
Table 6 Channel section geometry
The slope of the channel is obtained from the Equation 2.4 as

3.1 Design Formulas for Intakes
3.2 Design Formulas for Inlets
3.3 Design Formulas for Outlets
3.4 Design Formulas for Culvert
3.5 Design Formulas for Vertical Falls
3.6 Design Formulas for Spillways
3.7 Design Formulas for Siphon
Figure 2. Open intake

(A) Free discharge formula
|
|
m3/sec |
(3.1) |
where
f = velocity coefficient
Hl= contracted water depth, m
b = width of the gate, m= upstream energy level, m
H = upstream water depth, m
v0 = approach velocity, m/sec
g = 9.81 = acceleration due to gravity, m/sec2
Table 7 Values of Velocity Coefficient f
|
Types of gate |
f |
|
Broad crested gate |
0.85 - 0.95 |
|
Uncrested gate |
0.95 - 1.00 |
Contracted water depth:
|
H1 = y ×a |
(3.2) |
where f = contraction coefficient.
Table 8 Values of Contraction Coefficient y
|
|
y |
|
y |
|
y |
|
y |
|
0.00 |
0.611 |
0.30 |
0.625 |
0.55 |
0.650 |
0.80 |
0.720 |
|
0.10 |
0.615 |
0.35 |
0.628 |
0.60 |
0.660 |
0.85 |
0.745 |
|
0.15 |
0.618 |
0.40 |
0.630 |
0.65 |
0.675 |
0.90 |
0.780 |
|
0.20 |
0.620 |
0.45 |
0.638 |
0.70 |
0.690 |
0.95 |
0.835 |
|
0.25 |
0.622 |
0.50 |
0.645 |
0.75 |
0.705 |
1.00 |
1.000 |
(B) Submerged discharge formula
|
|
, m3/sec |
(3.3) |
where
C = f.y = discharge coefficient
a = opening height of the gate, m
b = width of the gate, m
h = Ho - H2 = head, m
Ho= upstream energy level, m
H2= downstream water depth, m
g = 9.81, m/sec2
The subcritical conjugate depth:
|
|
, m |
(3.4) |
where
H1 = contracted water depth
|
|
(3.5) |
|
|
(3.6) |
(C) Discharge formula influenced by downstream
|
Qretarded = k.Q, m3/sec |
(3.7) |
where
k = coefficient (Figure 3)
Q = free discharge, m3/sec (see Equation (3.1))
Figure 3. Values of coefficient k

Figure 4. The range of downstream influence on flow under gates

(D) Apron and floor length formulas
Total length of the apron:
|
|
(3.8) |
where
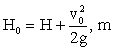
ds = depth of the sill from the downstream floor, m
4 h2 = length of the hydraulic jump, m
h2 = subcritical conjugate depth, m
Bligh's method
Total length of the floor:
Lf = C × HFS-b, m
where
C = Bligh's coefficient
HFS-b = difference between the full upstream supply level and the downstream bed level of the channel
Table 9 Values of Bligh's Coefficient C
|
Type of soil |
C |
|
Soft clay and silt |
3.0 |
|
Medium clay |
2.0 |
|
Loam |
5.0 |
|
Light sand and mud |
8.0 |
|
Peat |
9.0 |
|
Coarse grained sand |
12.0 |
|
Fine micaceous sand |
15.0 |
Example 3
A 3.0 m wide vertical uncrested gate discharges into a feeder channel in which the water level is 1.2 m. The upstream water level is 2.0 m and the gate opening is 0.70 m. The approach velocity is 0.75 m/sec. Determine the discharge through the structure and the length of the required apron.
By Figure 4 we find the type of flow condition existing

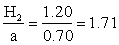

Observing the location of the point described, we note that the outflow is free. Therefore the free discharge is obtained using Equation (3.1)
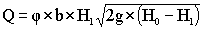
where from Table 7
f = 0.97 and

from Table 8
y = 0.628
then
H1 = y ×a = 0.628×0.70 = 0.44 m
therefore

Total length of the apron is defined from Equation (3.8)

where
H0 = 2.03 m
ds = 0
H1 = 0.44 m


then
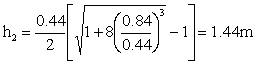
therefore

Figure 5. Pipe intake

Calculating formulas
|
|
m3/sec |
(3.9) |
where
C = discharge coefficient
A = pipe cross-sectional area, m2
h = head, difference in upstream and downstream water surface levels, m
Value of the discharge coefficient C is obtained by the formula
|
|
(3.10) |
where
ke =0.5 = entrance friction coefficient
l = 0.03 = friction factor for concrete pipe
l = pipe length, m
D = pipe diameter
Protection length on the downstream side is determined from the following formula:
|
|
(3.11) |
where
vp = velocity in pipe, m/sec
vs = scouring velocity, m/sec (Table 3)
Example 1
Determine the discharge of the intake and the required protection length on its downstream side with the data below
D = 45 cm
l = 12.5 m
Ho = 2.0 m
H2 - 1.6 m
Bed soil = sandy loam
Solution
Determine discharge from the formula (3.9)

where


h = H0-H2 = 2.0-1.6 = 0.4 m

From Table 3 the allowable velocity is defined as vs = 0.8 for sandy loam.
Then

therefore

3.2.1 Free fall pipe inlet
3.2.2 Submerged pipe inlet
3.2.3 Open flume inlet
Figure 6. Free fall pipe inlet

|
|
(3.12) |
where
Q = discharge of the inlet, m3/sec
m = discharge coefficient
A = internal cross-sectional area of the pipe, m2
g = 9.81 = acceleration due to gravity, m/sec2
H = head of the upstream water surface over the centre of the pipe, m
Figure 7. Discharge coefficient m for flow through free fall pipe inlet

Example 2
Determine the discharge of the free fall inlet with a diameter of 15 cm if its length is 4.0 m and the water depth in the feeder channel is 50 cm.
Solution
Determine the discharge from Equation (3.12)

The discharge coefficient is defined as m = 0.7 from Figure 7


then

Calculating formulas given for pipe intake may be used.
Figure 8. Open flume inlet l/sec


where
Q = design discharge of the inlet, l/sec
B = width of the throat, cm
H = height of the designed full supply level in the feeder channel above the sill level of the inlet
C = discharge coefficient
Table 10 Values of Discharge Coefficient C for open Flume Inlet
|
B in cm |
C |
|
6 to 10 |
0.0160 |
|
10 to 15 |
0.0164 |
|
over 15 |
0.0166 |
Figure 9. Relationships of discharge to B and H
|
Length of the cistern: |
lc = 0.82 H2, m | |
|
Depth of the cistern: |
dc = 0.1 H2, m | |
|
|
where |
H2 = water depth in the pond, m |
|
Depth of the glacis |
dg = 0.5 H, m | |
|
|
where |
H = maximum water depth above the sill level of the inlet, m |
Example 3
Design an open flume inlet for a discharge of 150 l/sec if the water depth in the feeder canal is 45 cm and 1.50 m in the pond.
Solution
The corresponding width of the throat to the water depth of 150 l/sec is defined from Figure 9.
then
B = 30 cm
dg = 0.5×H = 0.5×0.45 = 0.23 m
lc = 0.8×H2 = 0.8×1.50 = 1.20 m
dc = 0.1×H2 = 0.1×1.50 = 0.15 m
1. Open outlet (sluice)
2. Pipe outlet
Formulas given for intakes can be used.
The insertion of the stoplogs into the outlet creates over-shot flow conditions. The discharge formula (neglecting the approach velocity), for over-shot flow is:

where
B = internal width of the outlet monk, m
H = head, the difference between the pond water level and the stoplog crest, m
Time for emptying ponds or tanks:
|
|
(3.13) |
where
T = emptying time in seconds
A1 = average cross-sectional area of the pond or tank, m2
A2 = cross-sectional area of the outlet, m2
H1 = water depth in the pond at the beginning, m
H2 = water depth in the pond at the end, m
If the pond is completely emptied H2 will be = 0
Example 4
Determine the emptying time of 2 ha pond having its water depth of 1.5 m if the diameter of the outlet is 45 cm.
Solution
To obtain the required emptying time we use Equation (3.13)

where
A1 = 2 ha = 20 000 m2
H1 = 1.5 m
H0 = 0
then

Figure 10. Culvert

A) Submerged discharge formula
The formulas given for pipe intake can be used, but the following entrance friction coefficients should be used to calculate the discharge coefficient C.
Table 11 Values of Entrance Friction Coefficients for Culverts Flowing Full
|
Entrance condition |
ke |
|
Sharp-edged projecting entrance |
0.9 |
|
Flush entrance, square edge |
0.5 |
|
Well rounded entrance |
0.08 |
B) Unsubmerged discharge formula
|
|
(3.14) |
where
Q = design discharge of the culvert, m3/sec
A2 = actual flow area of the culvert, m2n = roughness coefficient for concrete pipe = 0.012 for corrugated metal pipe = 0.024
Pw = wetted perimeter of the culvertS = slope of the culvert
Entrance head:
|
|
(3.15) |
where
H = head on entrance above the bottom of the culvert, m
dc = water depth in the culvert, m
To solve Equation (3.15), it is necessary to try different values of dc and corresponding values of R until a value is found that satisfies the equation. If the head on a culvert is high, a value of dc less than the culvert diameter will not satisfy Equation (3.15). This means the flow is under pressure and discharge can be calculated by submerged discharge formula.
Figure 11. Vertical fall

A) Free discharge for vertical fall with a trapezoidal cross-sectional area
|
|
(3.16) |
where
Q1= design discharge of the vertical fall, m/secA1= contracted cross-sectional area of the flow, m v
H = upstream water depth in the channel, m
v = approach velocity, m/sec
H1 = contracted depth = 0.92 hc, m
h2 = critical depth, m
The critical depth can be obtained from the formulas (3.5) and (3.6).
B) Submerged discharge
|
|
(3.17) |
where
A = cross-sectional area of the flow, m2
hs = H2 - p, m
H2 = downstream water depth, m
ps = height of sill over-downstream bed level, m
h2 = subcritical conjugate level = 1.14 hc
C) Length and depth of the basin
The length of the basin is given by empirical formula
|
Lb = 5 (H×h)1/2 , m |
(3.18) |
where
H = upstream water depth, m
h = head, difference in upstream and downstream water surface levels, m
The depth of the basin is
|
db = 0.17 (H×h)1/2, m |
(3.19) |
3.6.1 Recommended design floods for the spillways
3.6.2 Types of spillways
3.6.3 Discharge formulas
|
Reservoir |
Design flood | |
|
Volume |
Height of dam |
(m/sec) |
|
(m3) |
(m) |
|
|
105 |
or max. 2.5 |
Q2% |
|
105 - 106 |
2.5 - 6.0 |
Q1% |
|
106 - 3.106 |
6.0 -10.0 |
Q0.5% - Q0.1% 1/ |
1/ In case the failure of the dam would create danger to human life or would cause great property damage, Q0,1% has to be used to design the spillway
1. Side channel spillways
1.1 Side earthen channel spillway2. Overfall spillway
1.2 Side lined channel spillway
3.1 Circular crest4. Siphon spillway
3.2 Standard crest
3.3 Flat crest
3.6.3.1 Side earthen channel spillway
Figure 12. Side earthen channel spillway

A) Discharge over the crest
|
|
(3.20) |
where
L = overflow crest length, m
h = water depth above the crest, m
Q = designed discharge, m3/sec
B) Velocity in the earthen channel
Using Manning's formula (2.1):
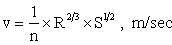
To prevent erosion in the earthen channel the calculated velocity should be less than the scouring velocity of the material concerned as shown in Table 3.
C) Length of the crest protection
|
|
(3.21) |
where
v = velocity of the designed discharge, m/sec
vs = scouring velocity, m/sec
h = water depth above the crest, m
3.6.3.2 Side lined channel spillway
The side channel has to be lined when the valley side has such great gradient that the calculated velocity in the side channel is higher than the scouring velocity concerned.
Example 5
Design a side earthen channel spillway for a discharge of 12 m3/sec in stiff clay soil if the gradient of the valley side along the axis of the channel is 4 percent.
Solution
The scouring velocity of stiff clay soil is defined as vs =1.4 m/sec from Table 3.
Considering the channel as an unkempt artificial channel with considerable aquatic growth the value of n equals to 0.030 from Table 2. The next step is to determine the measurements of the spillway.
Assuming that the water depth over the crest is h = 0.30 m the length of the crest is determined by the use of Equation (3.20).

For the length of the crest protection using Equation (3.21)
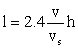
where

vs = 1.4 m/sec
h = 0.30 m
Entering these values into Equation (3.21)

To check the velocity in the channel first the measurements of the channel are defined as follows:
The cross-sectional area of the channel is:
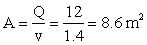
Assume that the channel has a bottom width of 30 m and its side slope of 1:1 then the normal water depth can be calculated by the following formula:
|
|
(3.22) |
where
z = ratio of the side slope (horizontal to one vertical)
with
b = 30 m
z = 1
A = 8.6 m2
then

The wetted perimeter is:

The hydraulic radius is
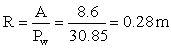
The velocity in the channel is then equal to

Since this velocity is higher than the scouring velocity, therefore, the channel should be lined or its gradient can be lowered by some falls. The slope of the bottom in the channel is obtained from Equation (2.1)

3.6.3.3 Overfall spillway
Figure 13. Overfall spillway

A) Discharge over the crest
|
Q = 1.7×L×h3/2, m3/sec |
(3.23) |
where
L = overflow crest length, m
h = water depth above the crest, m
Q = designed discharge, m
B) Design formulas for the glacis and the stilling basin
Critical depth is from Equation (3.5)

where

The velocity of the flow at the toe of the spillway may be computed by
|
|
(3.24) |
where
e = g×q
P = the crest height above the stilling basin
and
The head loss along the glacis can be determined by the formula
|
|
(3.25) |
where
n = roughness coefficient, sec/m1/3
1 = length of the glacis, m
The subcritical conjugate depth is defined by the formula
|
|
(3.26) |
The depth of the stilling basin is:
ds = h2 - h3, m
The length of the stilling basin can be calculated by the formula:
|
|
(3.27) |
Example 3.6
Design an overfall spillway with the following data
Q = 30 m3/sec
h = 1.0 m
P = 5.0 m
gradient of the glacis = 2:1
n = 0.012
h3 = 1.20 m
Solution
From Equation (3.23) the length of the crest is:

Discharge per unit width is

The critical depth is then

The length of the glacis is defined as
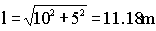
The head loss along the glacis is obtained from Equation (3.25)

where
Q = 30 m3/sec
n = 0.012
l = 11.18 m
A = hc×L = 0.66×18.0 = 11.88 m2
Pw = L + 2 hc = 18.0 + 2×0.66 = 19.32 m
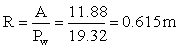
then

The velocity of the flow at the toe of the spillway is defined from Equation (3.24)

where

P = 5.0 m
hc = 0.66 m
h0 = 1.63 m

then


e = g×q = 9.81×1.67 = 16.38
now
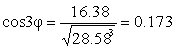



then

The water depth at the toe of the spillway is

The subcritical conjugate depth in the stilling basin is defined from Equation (3.26)

The depth of the stilling basin is
ds = h2 - h3 = 1.65 - 1.20 = 0.45 m
The length of the stilling basin is obtained from Equation (3.27)

where
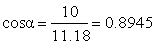
then

3.6.3.4 Shaft spillways
Figure 14. Types of the shaft spillways

(A) Discharge over the crest
1. Circular crest
|
|
(3.28) |
where
C1 = discharge coefficient (Table 12)
r = radius of the circular
h = water depth above the crest, m
Table 12 Values of the Discharge Coefficient C1,
|
h/r |
0.2 |
0.4 |
0.6 |
0.8 |
1.0 |
1.2 |
1.4 |
1.6 |
1.8 |
2.0 |
|
C1 |
1.82 |
1.78 |
1.63 |
1.33 |
1.12 |
0.93 |
0.80 |
0.70 |
0.62 |
0.57 |
2. Standard crest
|
Q = 2×C2×r×p ×h1/3, m3/sec |
(3.29) |
Table 13 Values of the Discharge Coefficient C2
|
h/r |
0.1 |
0.2 |
0.25 |
0.30 |
0.35 |
0.40 |
0.45 |
0.50 |
|
C2 |
1.83 |
1.825 |
1.815 |
1.80 |
1.785 |
1.76 |
1.74 |
1.72 |
3. Flat crest
|
Q = 3.2×r×p ×h3/2, m3/sec |
(3.30) |
3.6.3.5 Siphon spillway
Figure 25. Siphon spillway

|
|
(3.31) |
where
C = discharge coefficient
A = cross-sectional area of the throat, m
h = head, m
Value of the discharge coefficient is determined by the formula
|
|
(3.32) |
where
l = friction factor = 0.03 (concrete pipe)
1 = length of the siphon, m
d = diameter of the siphon, m
S k = all local loss coefficients (Table 19)
In order to determine the approximate size of the siphon the value of C can be considered as follows:
|
Types of siphons |
Diameter (mm) |
C |
|
medium |
120 - 200 |
0.4 - 0.6 |
|
large |
200 - 1 200 |
0.6 - 0.8 |
|
|
Diameter (mm) |
Length (m) |
Discharge (m3/sec) |
|
a) Small, mobile |
25 - 120 |
< 5 |
0.00025 - 0.015 |
|
b) Medium, movable |
120 - 200 |
< 10 |
0.015 - 0.050 |
|
c) Large, stabile |
200 - 1 200 |
< 100 |
0.050 - 3.10 |
Table 14 Recommended Minimum Velocities in Pipes for Siphons
|
Pipe diameter (mm) |
Velocity (m/sec) |
|
120 |
1.0 |
|
200 |
1.5 |
|
250 |
1.55 |
|
300 |
1.6 |
|
400 |
1.7 |
|
450 |
1.8 |
|
500 |
1.9 |
|
600 |
2.2 |
|
800 |
2.4 |
|
1 000 |
2.6 |
|
1 200 |
2.6 |
Figure 16. Details of the siphon

Calculating formulas
|
|
(3.33) |
where
C = discharge coefficient
A = cross-sectional area of the pipe, m2
H = head, m
The discharge coefficient C can be calculated by the formula
|
|
(3.34) |
where
l = friction factor = 0.02 (steel pipe)
1 = length of the siphon, m
d = diameter of the siphon, m
S k = all local loss coefficients along the siphon
Table 19 lists local loss coefficients for a variety of the fixtures.
The allowable pressure head for siphon
|
|
(3.35) |
where

|
Altitude in m |
0 |
500 |
1 000 |
1 500 |
2 000 |
3 000 |
|
|
10.3 |
9.8 |
9.2 |
8.6 |
8.1 |
7.2 |

|
Water temperature °C |
10 |
20 |
30 |
|
|
0.123 |
0.24 |
0.43 |
The allowable suction head of the siphon is:
|
|
(3.36) |
where
v = velocity in the pipe, m/sec

The maximum allowable downstream head of the siphon is:
|
|
(3.37) |
where

Depth of water above the entrance of the siphon
(a) Entrance with vertical axis
|
v |
|
D |
h | |
|
(m/sec) |
|
(m) |
(m) | |
|
1.5 |
0.1 |
- 0.3 |
2 D, but min. |
0.3 |
|
1.5 - 2.5 |
0.3 |
- 0.8 |
1 D |
0.7 |
|
> 2.5 |
> |
1.0 |
1.7 D |
2.0 |
(b) Entrance with horizontal axis
|
|
(3.38) |
where
ke = entrance loss coefficient
(c) Entrance with inclined axis
|
|
(3.39) |
where a = angle of the tilt in degree
Example 7
Design the siphon shown in Figure 17 for a discharge of 350 l/sec if water temperature is 30°C.
Figure 17. Details of the siphon

Solution
3 Considering the designed discharge Q = 0.35 m3/sec the siphon is a large one. The velocity is calculated by the following formula assuming that its diameter is 400 mm.

As this velocity is higher than the recommended minimum one in Table 14 hence, the selected diameter is satisfactory.
The next step is to determine the water depth above the entrance of the siphon by using Equation (3.38)
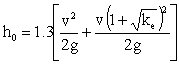
|
v = 2.79 m |
ke = 0.1 |
then
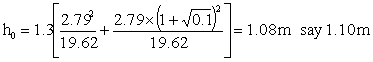
The discharge coefficient of the siphon is defined from Equation (3.34)

l = 0.02
l = l1 + l2 + l3 + l4 + l5 + l6 = 1.80 + 14.0 + 8.70 + 13.0 + 5.0 + 1.50 = 44 m
d = 0.40 m
Computation of the local loss coefficient using Table 19
|
Diffusor inlet |
0.1 |
|
Fraction bends (30°) |
4×0.09 = 0.36 |
|
Fraction bends (90°) |
0.34 |
|
Valve |
0.07 |
|
Outlet diffusor |
0.5 |
|
|
S k = 1.37 |
Substitution of the above values into the equation gives:
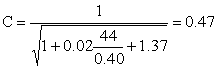
The allowable suction head of the siphon is obtained if we use Equation (3.35)

where


then

The suction head of the siphon is defined from Equation (3.36)

where
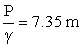

then
Hs = 7.35 - 1.03 = 6.32 m
Heffs = 550 - 545 - 5.0 m
The allowable downstream head of the siphon is determined from Equation (3.37)
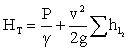
where
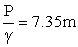

then
HT = 7.35 + 0.88 = 8.23 m
HeffT = 550 - 543 = 7.00 m
The design of the siphon is satisfactory because both Heffs and HeffT are below their allowable values.
The discharge of the siphon is defined by the formula (3.33)

where
C = 0.47
A = 0.126 m2
H = 545 - 543 = 2.0 m
then

This is acceptable, since the designed Q = 0.35 m3/sec.
4.1 Well Types
4.2 Well Discharge in a Confined Aquifer
4.3 Well Discharge in an Unconfined Aquifer
4.4 Radius of Influence
4.5 Screen Entrance Velocity
4.6 Recommended Well Diameter
Figure 18. Generalized cross section defining well types

Figure 19. Radial flow in a confined aquifer

Thiem's method
|
|
(4.1) |
where
k = permeability coefficient, m/sec
b = thickness of the aquifer, m
h0 = original piezometric head at the well
hw = piezometric head at the well, m
r0 radius of the influence, m
rw = radius of the well, m
Figure 20. Radial flow in an unconfined aquifer
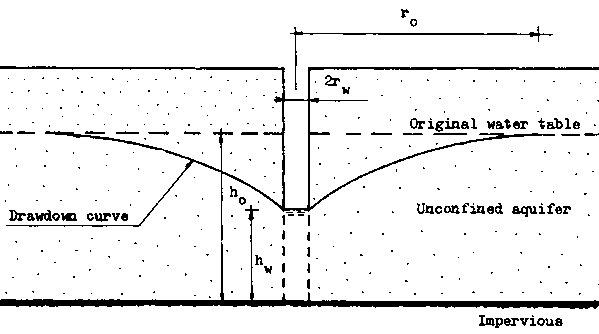
Dupuit's method
|
|
(4.2) |
|
|
(4.3) |
where
s = drawdown = h0 - hw, m, m
To ensure a long service life of the well, movement of the finer fractions of the aquifer material, resulting in subsequent clogging of the screen openings, has to be minimised. Therefore, the screen entrance velocities have to be kept below the values recommended in Table 15.
Table 15 Permissible Screen Entrance Velocities (Walton, 1962)
|
Permeability coefficient (m/day) |
Screen velocity (cm/sec) |
|
> 250 |
6.1 |
|
250 |
5.6 |
|
200 |
5.1 |
|
150 |
4.3 |
|
100 |
3.5 |
|
50 |
2.0 |
|
20 |
1.5 |
|
< 20 |
1.0 |
In order to install the required pumping equipment properly in the well, the diameter of the well should be determined on the basis of the well discharge as recommended in Table 16.
Table 16 Recommended Well Diameter (Smith, 1961)
|
Pumping rate (m3/hour) |
Well diameter (m) |
|
30 |
0.15 |
|
60 |
0.20 |
|
120 |
0.25 |
|
300 |
0.30 |
|
450 |
0.35 |
|
600 |
0.40 |
Example 1
Determine the discharge of a well with the diameter of 20 cm and the length of the screen of 30 m if k equals 10 m/day, the thickness of the unconfined aquifer is 40 m and the water table is at the depth of 6 m below the ground level.
In order to determine the well discharge the approximate value of drawdown is chosen as 4.0 m.
From Equation (4.3) we get

where
s = 4.0 m
k = 10 m/day = 1.2×10-4 m/sec
hence
From Equation (4.2) we get
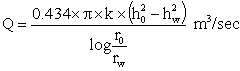
where
h0 = 34 m
hw = 30 m
rw = 0.10 m
Substitution of these values into Equation (4.2) gives
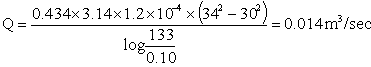
Determination of the entrance velocity of the screen:
The open area of the screen is assumed as 15 per cent of the total surface area of the screen. Then the screen entrance area is obtained by
As = 0.15×2rw×p ×Ls
where
Ls = 30 m
now
As = 0.15×2×0.10×3.14×30 = 2.82 m2
The effective open area accounting for blockage by grains is estimated to be 50 per cent of the actual open area i.e. 1.41 m . Hence the entrance velocity for a discharge of 0.015 m/sec is defined as
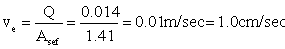
Since ve is equal to the optimum screen velocity, the selected screen is adequate.
Diameter of well:
Checking the well discharge per hour
q = 3 600×Q = 3 600×0.014 = 51 m3/sec
As this value is almost equal to the pumping rate of 60 m3/sec, hence, the selected diameter of 20 cm is adequate.
Example 2
Design a well for an indoor hatchery with a peak discharge of 700 1/min in an unconfined aquifer of 20 m. The fluctuation of the water table level is 4.0 m with a maximum level of 3.0 m below the ground level. Assume the value of k as 100 m/day.
(i) Selection of well diameterQ = 700 l/min = 42 m3/hour = 0.012 m3/secFor this discharge the recommended well diameter is 2rw = 0.15 m from Table 16.
(ii) Screen length
For k = 100 m/day = 1.16×10-3 m/sec the permissible screen entrance velocity is obtained from Table 15 as ve =3.5 cm/sec = 0.035 m/sec. The screen length is calculated from the formula below (Garg, 1978).Q = ve×Asef
where
Asef = effective open area of the screen = 0.5×0.15×As m2
Assuming that the screen's open area of 15 per cent is blocked by 50 per cent due to obstruction by aquifer grains
then
Substituting the values into the formula gives
(iii) Radius of influence
From Equation (4.3) we get
Assuming s = 1.5 m then

(iv) Discharge of the wellFrom Equation (4.2) we get

where
k = 1.16×10-3 m/sec
h0 =13 m, considering the minimum water table level
hw =11.5 m
r0 =153 m
rw 0.075 m
Substitution of these values into Equation (4.2) gives

Since the calculated discharge is larger than the required one, the above calculations have to be repeated with a lowered value of the drawdown.
Assume s = 1,0 m
then

This is equal to the peak water demand of the hatchery, hence the tube well with a diameter of 15 cm and a screen length of 10 m as well as a drawdown of 1.0 m yields the required 700 1/min for the hatchery.
Figure 21. Head loss in screens, values of screen loss coefficient Ks for various bar shapes) Mosonyi, 1963)

Kirschmer's formula
|
|
(5.1) |
where
hs = loss of head, m
Ks = screen loss coefficient
t = thickness of bars, m
b = clear spacing between bars, m
v = velocity of approach, m/sec
a = angle of bar inclination, degree
Example 1
Design a Screen chamber for a pumping station with the following data
|
Q |
= 0.50 m3/sec |
a = 70° |
|
h |
= 0.80 m |
|
|
v |
= 0.70 m/sec |
|
|
t |
= f 10 mm |
|
|
b |
= 2 cm |
|
Procedure:
(i) Head loss of screenHead loss of screens is obtained from Equation (5.1) with
Ks
= 1.79
t
= 0.01 m
b
= 0.02 m
v
= 0.70 m/sec
sin a
= sin 70° = 0.9397
Substituting these values into Equation (5.1) gives

(ii) Width of the pumping chamber
The width of the pumping chamber is calculated first without any screens as below

the number of spacing

now
t + b = 0.01 + 0.02 = 0.03 mHence, the total width of the screen chamber is obtained as
wef = ns×(t +b) = 45×0.03 = 1.35 m
Figure 22. Flow through filter

Darcy's Formula (Morris, 1963)
|
|
(6.1) |
where
Q = discharge of the filter, m3/sec
k = permeability coefficient, m/sec
h = head, m
L = thickness of the filter media, m
A = surface of the filter, m2
Table 17 Permeability Coefficient k
|
Soil type |
Average grain size (mm) |
Range of k (m/sec) |
|
Medium gravel |
4-7 |
(2.5 - 4.0)×10-2 |
|
Fine gravel |
2-4 |
(1.0 - 2.5)×10-2 |
|
Coarse sand |
0.5 - 2 |
10-4 - 10-2 |
|
Medium sand |
0.3 - 0.5 |
5.0×10-5 - 10-4 |
|
Fine sand |
0.1 - 0.3 |
(1.0 - 5.0)×10-5 |
Example 1
Design a filter box of a feeder channel against trash fish for a discharge of 200 l/sec. The thickness of the filter gravel with average grain size of 7 mm is 35 cm and the head is 30 cm.
Solution
Assuming that the length of the filter box is l = 3.0 m
From Equation (6.1)


where
A = l×w = 3.0m
L = 0.35 m
Q = 0.2 m3/sec
From Table 17 for average grain size of 7 mm we obtain k = 4.0×10-2
h = 0.30 m
then

so

Therefore, the required width of the filter box with the selected length of 3.0 m shall be 2.0 m.
Calculating Formulas
|
|
(7.1) |
|
|
(7.2) |
|
|
(7.3) |
where
Q = design discharge of the pipe, l/sec
S = H/L = slope of the energy line, m
H = head, m
L = length of the pipe, m
V = velocity in the pipe, m/sec
M = velocity modulus, m/sec
K = conveyance factor of the pipe, l/sec
Table 18 Velocity Moduli and Conveyance Factors of the Pipes

Minor losses along the pipe may be expressed in the equivalent length of pipe that has the same head loss for the same discharge. The chart in Fig. 23 shows a convenient method of estimating these losses
Example 1
Determine the discharge of a 200 mm diameter galvanized pipe if the length of the pipe is 1 000 m and the head loss is 5.0 m.
Solution
The above given conditions imply that:
L = 1 000 m
D = 200 mm
H = 5.0 m
Figure 23. Minor losses of valves and fittings to flow of water (Coronel, 1978)

From Equation (7.1)

from which
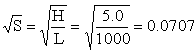
From Table 18 K for the galvanized pipe of 200 mm in diameter is 476.9 l/sec.

Example 2
Determine the required head loss for a discharge of 50 l/sec in the pipe described in Example 1.
Solution
Using Equation (7.2) modified below

where
L = 1.0 km
From Table 18

Then
H = 502×1.0×0.0044 = 11.0 m
In the hydraulic design of pipelines the energy loss through friction along the pipe is dominant for pipes of 50 m or longer. For shorter pipe lengths the aggregate of local energy losses at elbows, valves, inlet devices etc., may be equal or more than the frictional losses along the pipe. Local losses in piping fixtures were found to be proportional to the amount of kinetic energy entering the fixture. The configuration of the fixture determines the constant of proportionality. Accordingly, local loss in a pipe fixture is computed by

in which k is the so-called local loss coefficient and v is the velocity in the pipe before the fixture, unless otherwise specified. Table 19 lists local loss coefficients for a variety of fixtures.
Table 19 Local loss coefficients

Table 20 Recommended Velocities in Pipes for Water Supply
|
Pipe diameter (mm) |
Velocity (m/sec) |
Pipe diameter (mm) |
Velocity (m/sec) |
|
25-50 |
0.60 |
400 |
1.25 |
|
60 |
0.70 |
500 |
1.40 |
|
100 |
0.75 |
600 |
1.60 |
|
150 |
0.80 |
800 |
1.90 |
|
200 |
0.90 |
900 |
1.95 |
|
250 |
1.00 |
1 000 |
2.00 |
|
300 |
1.10 |
1 200 |
2.20 |
8.1 Types of Pumps Used in Aquaculture
8.2 Total Dynamic Heads
8.3 Specific Speed
8.4 Net Positive Suction Head (NPSH)
8.5 Power Requirement
8.6 Determination of the Most Economical Pipe Diameter
1. Propeller pumps
2. Centrifugal pumps
3. Turbine pumps
Figure 24. Details of a pump station

Calculating formulas (Hicks, 1957)
|
HT = HST + HDT = total dynamic head, m |
(8.1) |
where
HST = He + HSS +HSV + HSf = tota1 suction head, m
HDT = HDS + HDV + HDf = total discharge head, m

ke = local loss coefficient of the mouthpiece (Table 19)
vs = suction velocity in the suction pipe, m/sec s
HSS = suction static head is the vertical distance in metre between the downstream water surface and the centreline of the pump. It may be either positive or negative, depending upon the location of the pump centreline with respect to the water surface
 suction velocity head is the equivalent head through which the water would have to fall to acquire the velocity it has in the suction.
suction velocity head is the equivalent head through which the water would have to fall to acquire the velocity it has in the suction.
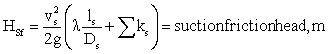
l = 0.02 = friction factor (steel pipe)
ls = length of the straight suction pipe, m
Ds = inside diameter of the suction pipe, m

S ks = local losses of the suction pipe, m
Q = discharge of the pump, m3/sec
A = pipe cross-sectional area, m2
HDS = discharge static head is the vertical distance in metre between the centerline of the pump and the point of discharge

vd = velocity of flow in the discharge, m/sec
vs = velocity of flow in the suction, m/sec
If the suction and discharge openings are of equal diameter, the discharge velocity head will be zero.

ld= length of the straight discharge pipe, m
Dd = inside diameter of the discharge pipe, m
S kd = local losses of the discharge pipe, m
|
|
(8.2) |
where
n = impeller speed, rpm
Q = discharge, m3/sec
H = head, m
Specific speed n is a widely used criterion for pump selection. It is the impeller speed corresponding t §a discharge of 1.0 m3/sec at 1.0 m of head for the most efficient design. The recommended design range of ns is shown in Table 24.
Table 24 Recommended Design Range of ns
|
Type of pump |
ns | |
|
Centrifugal pumps radial flow with |
| |
|
|
narrow impeller |
10 - 30 |
|
|
medium impeller |
30 - 45 |
|
|
wide impeller |
45 - 80 |
|
Centrifugal pumps mixed flow |
80 -150 | |
|
Propeller pumps |
135 -320 | |
|
|
(8.3) |
where


To prevent cavitation, the pump should be placed such that the total suction head HST is less than the head available, based on the local atmospheric pressure minus the vapour pressure of the water.
Power required by a pump motor is commonly expressed in terms of brake horsepower and may be computed as follows:
|
|
(8.4) |
where
g = 1 000 kg = unit weight of water in m
Q = discharge of the pump, m3/sec
HT = total dynamic head, m
h p = efficiency of the pump
h m = efficiency of the motor
Efficiency of a pump varies with Q and H. The value is included in the manufacturer's characteristic curves of the pumps available in pump catalogues, an example of which is shown in Figure 25.
Figure 25. Characteristic curves for pump AGROFIL 500-D
The electric motor's power requirement must be expressed in kilowatts
1 horsepower = 0.7457 kW
Therefore, if Equation (8.4) is expressed in kilowatt we obtain:
|
|
(8.5) |
Efficiency of the motor depends upon the type of the driven motors as follows:
|
Types of the driven motors |
h m |
|
Direct-coupled electric motor |
0.90 - 0.95 |
|
Diesel engine |
0.65 - 0.80 |
To ensure the minimum operation cost and the amortization of a pump station having a longer pipeline, its most economical pipe diameter can be defined by the following function as Agroszkin (1952) recommended.
|
|
(8.6) |
where
g = 1 000 kg = unit weight of water in m
Q = discharge of Q the pump, m3/sec
T = yearly pumping hours
Rc = unit cost of a horsepower-hour
h = h p×h m = total efficiency
pa = percentage of the amortization
Cp = unit cost of one metre diameter pipe per metre
The values of function FD are shown in Table 22.
Table 22 Values of Function FD
|
Diameter |
|
Types of pipes |
|
|
(inches) |
(mm) |
Plastic, G.I. new cast iron |
Concrete |
|
2 |
50 |
0.000002 |
0.000001 |
|
3 |
75 |
0.00002 |
0.000013 |
|
4 |
100 |
0.00012 |
0.000082 |
|
5 |
125 |
0.00049 |
0.00033 |
|
6 |
150 |
0.0015 |
0.00105 |
|
7 |
175 |
0.0040 |
0.0028 |
|
8 |
200 |
0.009 |
0.013 |
|
9 |
225 |
0.019 |
0.01364 |
|
10 |
250 |
0.036 |
0.026 |
|
12 |
300 |
0.114 |
0.081 |
|
14 |
350 |
0.296 |
0.213 |
|
16 |
400 |
0.679 |
0.490 |
|
18 |
450 |
1.409 |
1.022 |
|
20 |
500 |
2.71 |
1.97 |
|
24 |
600 |
8.4 |
6.2 |
|
28 |
700 |
21.9 |
16.1 |
|
30 |
750 |
33.5 |
24.8 |
|
32 |
800 |
50.0 |
37.0 |
|
36 |
900 |
103.6 |
76.9 |
|
40 |
1 000 |
199.1 |
148.3 |
Example 1
A pump with the designed arrangement as shown in Figure 24 delivers 175 l/sec. Determine the total dynamic head and the required brake horsepower.
Solution:
The total dynamic head is obtained by Equation (8.1)
HT = HST + HDT
where
HST = He + HSS + HSV + HSf,
and
HDT = HDS + HDV + HDf
The first step is to determine the various heads which are computed as follows:

From Table 19 for strainer bucket without foot valve
ke = 5.5
From Figure 24
D = 300 mm

then

hence

HSS = 4.0m


ls = 2.5 + 4.0 = 6.5 m
l = 0.02
D = 0.30 m
kb = 0.17 (45° bend. Table 19)
Substituting these values in Equation HSf we get

The total suction head is, therefore
HST = 1.68 + 4.00 + 0.305 + 0.184 = 6.169 m
From Figure 24
HDS = 3.0 m
As the discharge and suction pipes have the same diameter, hence
HDV = 0

lD= 3.0 + 28.0 + 1.0 = 32.0 m
From Table 19 for gate valve with e/D = 1/3
kv = 0.07
for two 90° bends with R/d = 1
kb = 2×0.53 = 1.06
then

The total discharge head is, hence
HDT = 3.0 + 0 + 0.995 = 3.995 m
Then the total dynamic head is
HT = 6.169 + 3.995 = 10.164 m say 10.20 m
The brake HP is determined by the use of Equation (8.4)
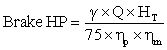
where
g = 1 000 kg
Q = 0.175 m3/sec
HT = 10.20 m
Assuming
h p =0.75 and h m =0.90
then

Example 2
Determine the most economical pipe diameter for the pump station described in Example 1 with the following data
T= 20.365 = 7 300 hours a year
Rc = US$ 0.07
pa = 10%
Cp = US$ 175
Using Equation (8.6) with the above data we obtain

From Table 22, the corresponding diameter is defined as
D = 500 mm