4. MEASURING VERTICAL ANGLES AND SLOPES
4.0 Introduction
|
|
1. A vertical angle is an angle formed by two connected
lines in the vertical plane*, that is, between a low point
and two higher points. Since these angles are in the vertical plane,
the lines that form them will usually be lines of sight. A vertical
angle BAC can be formed, for example, by the line of sight AB
from station A on a river bank to a higher water-pump installation,
and the line of sight AC from station A to a much higher water-storage
tank.
2. Whenever a line is not horizontal, it has a slope. The
slope can be uphill or downhill. Its steepness depends on the difference
in height between its points.
3. As you have learned (see Chapter 2), the
slope of the ground affects the measurement of distances. Ground
slope is also very important in the design of fish-farms, since you
can use it to reduce your construction costs. You need to
build bottom slopes in canals, to allow the water to move by gravity*;
and in ponds, to allow good drainage. And you must build slopes in the
dikes for ponds and dams (see the next manual in this series, Constructions
for Freshwater Fish Culture).
|
|
|
|
4. The slope of a line is called the gradient. It may be
defined as:
- The change in vertical distance or elevation* over a
given horizontal distance, or the change in horizontal distance
over a given vertical distance;
- The vertical angle made by the sloping line and a horizontal
line.
|
|
|
| |
|
|
|
|
|
|
| |
|
|
5. The slope of a line is therefore expressed in various
ways:
- as a percentage, or the number of metres of change in
elevation over a horizontal distance of 100 m. This may be written
in two ways, either as a percent (%) or as a decimal value, in hundredths;
|
|
|
| |
|
|
- in degrees, as the measurement of the vertical angle
made by the slope and the horizontal plane*.
Remember that:
- degrees are subdivided into 60 minutes (60'), each minute
equalling 60 seconds (60");
- a right angle equals 90�, and therefore a slope is always measured
between 0� (horizontal) and 90� (vertical);
|
|
|
| |
|
|
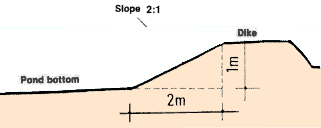
- As a ratio,
showing the change in horizontal distance (x) per unit of vertical
distance, or the change in vertical distance (y) per unit of horizontal
distance, in one of the following ways:
- the change
in horizontal distance (x metres) per one metre of vertical distance;
this can express, for example, the slope of the sides of dikes and
canals (such as 2:1);
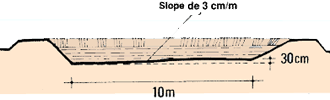
- the change
in vertical distance (x millimetres or x centimetres) per one metre
of horizontal distance; this can express, for example, the lengthwise
slope of a pond bottom or water pipe (such as 3 cm/m);
- the change
in horizontal distance (x units) per one unit of vertical distance.
This can express, for example, the lengthwise slope of a pipeline
(such as 1 in 300).
|
|

1 m change in elevation every 300 m
|
| |
|
|
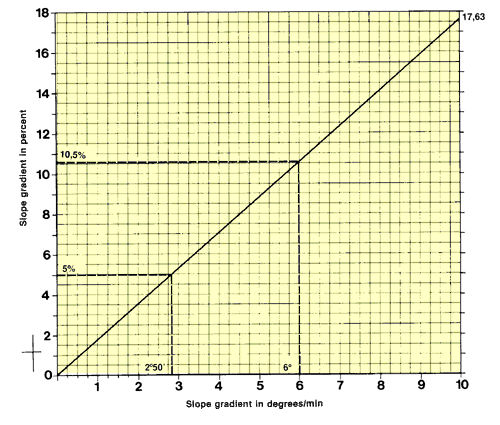
Converting percentage of a slope into degrees, or degrees into percentage
|
| 6. Depending on the instrument you are using to measure
a slope directly, you may sometimes have to convert the percentage
of the slope into degrees, or the degrees into percentage. For help with
such a conversion, you should use either Table 4 or the graph given in Figure 3. |
|
|
| |
|
|
|
Note: from the table and the graph you can see
that:
- 1 degree is about 1.75 percent;
- 1 percent is about 0�35';
- A 45� slope = a 100 percent slope.
|
|
|
|
TABLE 4
Conversion of slope units in degrees or percentages
From percent into degrees
| Percent |
Degrees/min/s |
|
0.5
|
0�17'10''
|
|
1
|
0�35'
|
|
2
|
1�08'40''
|
|
5
|
2�51'40''
|
|
10
|
5�42'40''
|
|
20
|
11�18'36''
|
|
30
|
16�42'
|
|
40
|
21�48'05''
|
|
50
|
26�33'55''
|
|
100
|
45�
|
|
|
From degrees into percent
| Degrees |
Percent |
Degrees |
Percent |
|
0.25(15')
|
0.44
|
11
|
19.44
|
|
0.50(30')
|
0.87
|
12
|
21.26
|
|
0.75(45')
|
1.31
|
13
|
23.09
|
|
1
|
1.75
|
14
|
24.93
|
|
2
|
3.49
|
15
|
26.79
|
|
3
|
5.24
|
16
|
28.68
|
|
4
|
6.99
|
17
|
30.57
|
|
5
|
8.75
|
18
|
32.49
|
|
6
|
10.51
|
19
|
34.43
|
|
7
|
12.28
|
20
|
36.40
|
|
8
|
14.05
|
30
|
57.74
|
|
9
|
15.84
|
40
|
83.91
|
|
10
|
17.63
|
45
|
100
|
Remember: 60 min = 1 degree and
60 s = 1 min
|
Examples:
-
a slope of 17 percent is equal to (10 + 5 + 2) percent, which is equivalent
to 5�42'40'' + 2�51'40" + 1�08'40" = 8�101'120'' = 8�103' = 9�43';
-
a slope of 9�43' is about equal to (9� + 30' + 15'), which is equivalent
to 15.84 percent + 0.87 percent + 0.44 percent = 17.15 percent or 17 percent.
FIGURE 3
Graph for the rapid conversion of slope units
|
Measuring and calculating slopes
|
|
7. There are two groups of methods for determining slopes.
|
|
Measuring slope directly
|
| |
|
|
|
- You can calculate the slope: measure the ground-level difference (in
metres) between two points along the steepest part of the slope (called
the axis), using one of the devices described
in Chapter 5. Calculate the slope, which you will usually express
as a percentage (see next step).
|
|
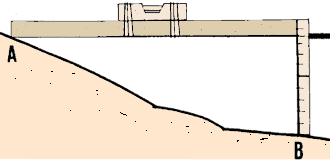
Measuring ground-level differences to calculate slope
|
| |
|
|
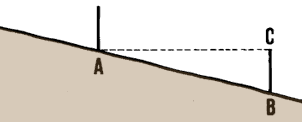
8. To calculate the slope, proceed as follows:
- along the axis of the slope, measure the difference in level
AC between two marked points A and B (see Chapter 5);
- measure the horizontal distance CB between points
A and B (see Chapter 2);
- calculate the slope S in percent as equal to:
|
|
First measure the difference in level
|
| |
|
|
|
Note: to make your calculations easier:
- you can fix the horizontal distance CB at 100 m, which
will give you S% = AC directly in metres;
- you can fix the horizontal distance CB at 10 m instead,
which will give you S% = 10 AC in metres.
Remember: you must measure the horizontal
distance!
|
|
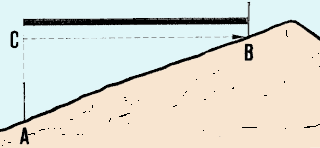 Then measure the horizontal distance
Then measure the horizontal distance |
Using slope to calculate horizontal distances
|
| 9. In Chapter 2, Sections 2.6 and 2.7, you learned that when
measuring a distance AB on sloping ground, you need to correct this measurement
in order to find the true horizontal distance AC, but only when
the slope exceeds 5 percent (or about 3 degrees). To make these
corrections, you may use either the method described below, or the
method which will be described in Section 5.0, step 17. To
calculate horizontal distances from distances measured over sloping ground,
proceed as follows: |
|
|
| |
|
|
| 10. Measure the distance AB (in metres) on the ground
between points A and B (see Chapter 2).
11. Measure the average ground slope S in
degrees between points A and B (see this chapter, Sections 4.1 to
4.7).
Note: if the slope is measured in percent, you
will have to convert it into degrees (see Table
4 or Figure 3).
|
|
Measure the ground-level distance 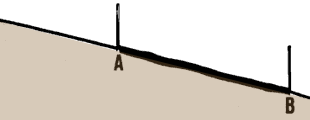
|
| |
|
|
|
12. Enter this average slope S (in degrees)
in Table 5 to obtain the value of cosine S (cos S). If
the slope does not correspond exactly to any of the angle values given
in the table, you will have to calculate cos S by using proportional
parts (see example in Table 5).
13. Calculate the horizontal distance AC (in metres) using
the formula:
|
|
Measure the slope
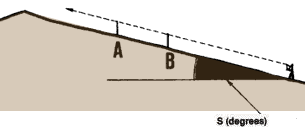
|
| |
|
|
Calculate the horizontal distance
|
|
AC = AB cos S

|
TABLE 5
Cosine values of angles (d = degrees, m = minutes, cos = cosine,
x = difference)
|
MAIN TABLE

|
|
TABLE OF PROPORTIONAL PARTS, P

Example
To calculate intermediate cosine values using the proportional
parts, for cos 7�38' for example, proceed as follows:
- from the Main Table, calculate cos 7�30' = 0.9914;
- obtain the difference between this value and the next, x = 3;
- find column 3 in Table of Proportional Parts, P;
- move down this column to line m = 8, to find P = 2.4;
- subtract P from the last number (4) of the value read from the
Main Table, 0.9914 - 0.00024 = 0.99116. This is cos 7�38'.
|
Choosing a method to use for measuring slopes
|
|
14. There are several good ways to measure slopes. The method
you use will depend on several factors:
- how accurate a result you need;
- the equipment you have available;
- the type of terrain on which you are measuring.
Each of the various methods is fully explained and illustrated in the
following sections, except for the method to use with levelling devices
(see Chapter 5). Table 6 will also help
you to compare the various methods and to select the one best suited to
your needs.
|
|
|
| |
|
|
Plumb-line
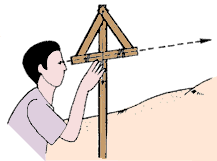
|
|
Clisimeter
|
|
TABLE 6
Vertical angle and slope measurement methods
|
Section1
|
Method 2
|
Accuracy
|
Remarks
|
Equipment
|
| 4.1*,4.2* |
Clinometer, models 1 & 2
|
Low
|
Quick and rough estimate
for rather steep slopes
Hand-held instrument
|
Home-made clinometer
|
| 4.3* |
Clinometer, model 3
|
Low to medium
|
To be fixed in ground
Direct reading in percent
|
Home-made clinometer
|
| 4.4* |
Clinometer, model 4
|
Low to medium
|
To be fixed in ground
Small, easy to make
Direct reading in percent
|
Home-made clinometer
|
| 4.5** |
Clisimeter
|
Low (about 10 percent)
|
Quick and rough estimate
Direct reading in percent
|
Lyra clisimeter
|
| 4.6** |
Optical clinometer
|
Medium to high
|
Quick, rather good estimate
Direct reading in degrees and percent
|
Optical clinometer
|
| 4.7*** |
Miscellaneous levelling devices
|
Medium to high
|
Requires distance measurement
Best estimates for small gradients, especially with best levels
|
|
1 *Simple **more difficult ***most difficult.
2 In italics, equipment you can build
yourself from instructions in this manual.
|
4.1 How to measure with the home-made clinometer, model 1
1. A clinometer is an instrument for measuring
slopes or vertical angles. There are various types of clinometers, but they
all include a graduated arc similar to a protractor (see Section
3.3, step 11). To use the clinometer, you hold it in your hand and read the
slope against this arc. You also usually refer to a free-hanging plumb-line
called the pendulum. There is a line of
sight* on the top of the clinometer. You can easily make your own
simple clinometer; four models are described in Sections 4.1 to 4.4.
Adjusting
your home-made clinometer
5. Measure the vertical distance from the level of your eyes to
the ground, then measure the same vertical distance on a wall and
mark it clearly. You will also need to mark this vertical distance
clearly on a pole or staff, which you will use for sighting.
- Get a straight pole whose length is equal to your eye
level plus 25 cm. (For example, if your eye level is 145 cm, the pole should
be 145 cm + 25 cm = 170 cm.) Make a sharp point at one end and drive it into
the ground until the top of the pole is at your eye level. Carefully mark
the point where the pole enters the ground, called the reference*
level; when you use the pole, always drive it in up to this line.
To make the top of the pole more visible, mark it with bright-coloured paint
or cloth. You will sight at the top of the pole.
Make a mark at your eye level

|
|
Mark the reference level on the pole
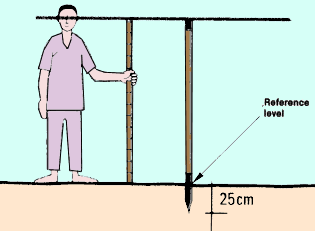
|
| |
|
|
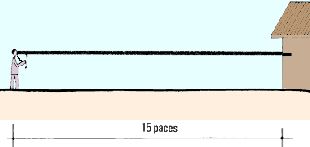
| 6. Stand on horizontal ground about
15 paces in front of the mark, and aim at it through the sighting device
on your clinometer. |
|
7. Check that the plumb-line string indicates 0�.
If it does not, adjust the small nail holding the plumb-line. When the
string indicates 0�, your clinometer is ready to use. |
| |
|
|
Check for accuracy by sighting at the mark you have made
|
|
|
Using your clinometer to measure a slope
8. Sighting either uphill or downhill with the clinometer,
you can measure a slope by moving the protractor around.
9. Take a position with the
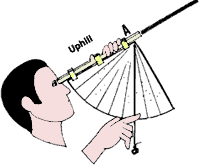
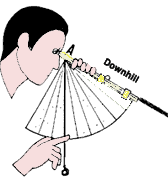
clinometer. Make sure to stand up straight so you do not change your eye level. Sight at a
point. This point should be:
- at eye level; use the pole or
staff you prepared in step 5, making sure that it is vertical;
- no farther away than 30 m - a
shorter distance (15-20 m) will improve the accuracy of the measurement.
|
|
|
Make sure point A is at the top,
whether you are sighting uphill or downhill
|
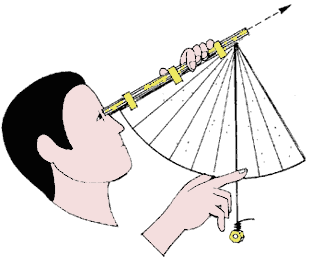
10. With the clinometer in sighting position, press the plumb-.line with your
finger against the bottom scale. Be careful not to move the plumb-line from
its vertical position. Read the scale at the point where the plumb-line intersects
the degree graduation. This reading is the slope, in degrees.
Note: you can convert
your degree measurement into a percentage (see Section 4.0).
Hold the plumb-line in place with your finger
|
|
|
4.2 How to measure with the home-made clinometer, model
2
1. You can make another type of clinometer from wood or metal. This model also
has a plumb-line, but its reference scale gives you the slope in percent.
Making the pendulum clinometer, model 2
2. Cut a 51 x 51 cm square board
from a piece of wood, or build one from strips of wood or metal.
- If you cut the board from wood, use a piece which is heavy enough to prevent
warping. Plywood or particle board 1.5 to 2 cm thick will usually be good
to use.
- If you build the board from wooden or metal strips, be sure the finished
board is square. Carefully join wooden strips at the corners. You may need
to brace them at the back with diagonal wooden strips. Securely weld metal
strips together.
|
|
|
Reinforce the board if necessary
|
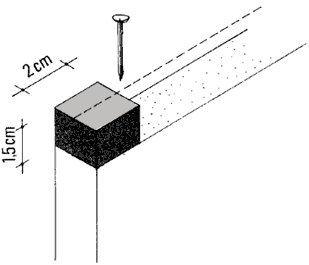
3. Provide a sighting line* along the upper edge of the square.
- If the board is made of wood, you can make a sighting line by driving a
finishing nail (small-headed) into the top edge of the board at a point 2
cm in from each vertical edge. To make sure the nails are at the same
height, place a block of wood 1.5 to 2 cm thick next to the first nail,
and drive the nail in so that it is even with the top of this block. Then
move the measuring block to the other end and use the same method for the
second nail.
- If the board is made of metal, you can make a sighting line by glueing or
welding two nails or metal points on the top edge of the board. Be certain
that the nails are at the same height.
|
|
A block of wood helps you place the nails correctly
|
| |
|
|
| 4. Provide a centre-point from which to hang a plumb-line.
Put a mark on the board 1 cm down from the top edge and 1 cm in from
the sighting point which is furthest from your eye. If the board is wooden,
drive a nail into this mark; if it is metal, weld a small nail to the mark,
or drill a hole through it. |
|
|
| |
|
|
| 5. Make a plumb-line about 65
cm long, using a piece of thin string and a weight. A plumb-bob (a small
lead weight) will make the best weight for the plumb-line but, if you do
not have one, you can use any object which has its weight evenly distributed
from a single point. A heavy nut or washer, or a wooden disk with a hole
in the centre, will work. |
|
|
| |
|
|
|
6. Attach the plumb-line to the hole or nail at the centre-point of the
board.
7. Loosely attach a ruler graduated in centimetres along the
bottom edge of the board; use large clips, or tie the ruler on with string.
Position the ruler so that its zero graduation is directly under the centre-point.
Make sure that the distance between the centre-point of the plumb-line
and the zero mark on the bottom edge of the ruler is 50 cm.
|
|
|
Adjusting your clinometer
|
| 8. Aim the board at a mark which you have aligned at eye level.
Standing straight and looking along the board's upper edge, align
the two sighting points with this mark. Your sighting line* should
now be horizontal and your plumb-line should be vertical. |
|
|
| |
|
|
|
9. Put your thumb on the plumb-line to hold it against the ruler at the
bottom of the board, and check to see if the line is at zero.
If it is not, adjust the position of the ruler so that the zero graduation
and the plumb-line fall exactly in line.
10. Check to see that your clinometer is correctly aligned by sighting
again. When it is, glue or nail the ruler firmly in place.
Your clinometer is now ready to use.
|
|
|
|
Using the clinometer to measure a slope
|
|
11. You can measure both uphill and downhill slopes with your clinometer
in the following ways:
|
| |
|
|
- To measure uphill slopes, the plumb-line should be at the edge of
the board furthest from your eye when you are sighting;
|
|
- To measure downhill slopes, the plumb-line should be at the edge of
the board nearest to your eye when you are sighting.
|
|
|
|
|
| |
|
|
| 12. Place a pole or a staff clearly marked at eye level
(see Section 4.1, step 5) on a point you can easily see, usually 15 to 20
m away. |
|
|
| |
|
|
| 13. Aim the clinometer at this mark and, when the plumb-line
has stopped swinging, press it with your finger to the ruler at the bottom.
Be careful not to move the plumb-line from its vertical position. Then,
read the graduation (in centimetres) at this point.
14. Since every centimetre on the ruler equals 2 percent of slope, calculate
the slope as a percentage by multiplying the number of centimetres
you read on the graduation by 2.
Example
If you read 2.5 cm on the ruler, the slope is found as:
2.5 cm x 2 = 5%
|
|
|
4.3 How to measure with the home-made clinometer, model
3
1. The third model of clinometer is a little more complicated to make, but
it is more accurate. It is also easier to use if you are measuring on ground
that is soft enough for you to drive in the supporting staff.
Making the clinometer, model 3
|
| 2. To make the supporting staff, get a straight
stick or a piece of wood about 2 m long. Shape one of its ends into a point,
so that you can easily drive it into the ground. About 25 cm from the pointed
end, mark a line to show how deep you will drive the staff in. |
|
|
| |
|
|
|
|
|
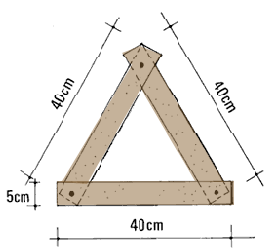
3. Get three pieces of wood exactly the same, 40 cm long, 4 to 5 cm wide,
and about 1 cm thick. Secure them tightly together with nails or screws
to form a triangle with three equal sides.
|
| |
|
|
| 4. Prepare a ruler graduated in millimetres.
Get a piece of wood about 25 cm long, 4 cm wide and 0.25 cm thick. Mark
the centre with 0, then mark graduations from this centre-point up to 100
mm on either side. |
|
|
| |
|
|
|
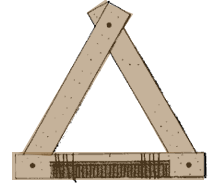
5. Loosely attach this ruler to one of the triangle's sides with string
or clips.
|
|
Tie the ruler to the triangle with string
|
| |
|
|
|
6. On the same side of the triangle, make a sighting device.
Drive two nails vertically into the side near each of its ends. Make sure
the nails are at equal heights and on the line.
|
|
|
| |
|
|
| 7. Drill a small hole exactly at the centre of the triangle's
summit, opposite the zero point of the ruler.
8. Attach the triangle near the top of the supporting staff with a nail;
make sure that the triangle remains free to swing around this axis.
9. Prepare a plumb-line about 40 cm long
(see Section 4.2). Attach it to the nail at the centre of the triangle's
summit.
|
|
Nail the triangle so it swings freely 
|
Adjusting the clinometer
|
|
10. Drive the supporting staff vertically into
horizontal ground until you reach the reference* level you
marked above its pointed end.
11. Measure the vertical distance between the ground and the sighting
line* of the clinometer exactly. This distance should
be about 130 cm. Prepare a pole or staff that shows this height
(see Section 4.1, step 5).
Note: the height of the sighting line for this
clinometer may be different from your eye level.
|
|
|
| |
|
|
| 12. About 15 paces away, make a mark on a wall set at the
same height you just measured. Aim with the sighting line at this mark.
|
|
Sight at the mark

|
| |
|
|
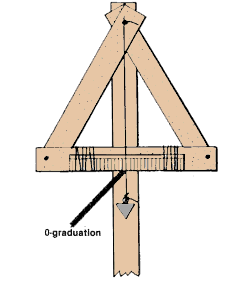
| 13. Adjust the position of the ruler so that its 0-graduation
lines up exactly with the plumb-line. Check again for sighting-line accuracy
and adjust the 0-graduation if you need to, then glue or nail the ruler
firmly in position on the triangle. The clinometer is now ready to use. |
|
14. Exactly measure the distance (in centimetres)
between the point at which the plumb-line is attached and the point where
the sighting line intersects the plumb-line. This distance should be about
32 cm, and is the standard distance D of your clinometer. Be
sure to measure D precisely. |
| |
|
|
Adjust the ruler so the plumb-line is at zero
|
|
Measure the distance D from the nail to the sighting
line
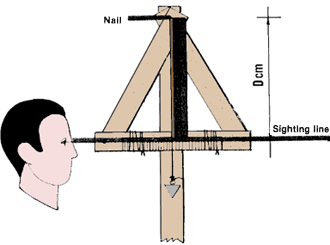
|
Using your clinometer to measure a slope
|
|
15. You can measure either uphill or downhill slopes by reading the
appropriate one of the two scales.
16. Place a pole or staff clearly marked at the sighting-line
level (see step 11) on a point B of the slope you are measuring,
about 15-20 m away.
|
|
17. At point A, drive your clinometer support vertically
into the ground, down to the reference level. With the sighting line,
aim at the mark on the pole or staff; to do this, slowly swing the triangle
around the nail at its top until you sight the marked level. |
| |
|
|
|
|
Swing the triangle around until you sight the
top
of the pole
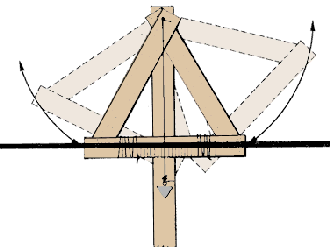
|
| |
|
|
| 18. When the sighting line is level with this mark, press
the plumb-line with your finger against the ruler. Be careful not to move
the plumb-line from its vertical position. |
|
19. Read the graduation N (in millimetres)
on the ruler at the point where the plumb-line intersects the sighting
line. |
| |
|
|
Hold the plumb-line in place
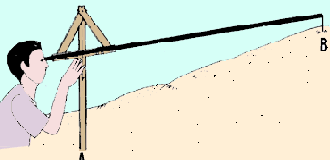
|
|
|
20. If the standard distance of the clinometer (see step 14) is
D (in centimetres), calculate the ground slope S% as:
Example
If D = 32 cm and you read a graduation of 4.8 cm = 48
mm on your clinometer, the slope is equal to:
(10 x 48) � 32 = 15%
4.4 How to measure with the home-made clinometer, model
4
1. The fourth clinometer model is similar in principle to the
preceding one, but it has several improvements: it is much smaller in size;
it is easier to make; and it provides a direct reading of the slope, so that
you do not need to make any calculations. The model 4 clinometer may also be
used to measure vertical angles (see this Section, step 17).
Making the clinometer, model 4
|
| 2. Get a small piece of thin wooden board, about 14 x 21 cm.
The best material would be plywood. |
|
3. On this board, glue a sheet of squareruled millimetric paper so that
its printed lines are parallel to the sides of the board.
4. Draw a line AB, parallel to the larger edge of the board and about
1.5 cm from it.
|
| |
|
|
|
|
|
|
| |
|
|
| 5. Find the centre of line AB and mark it C. From
this point lay out perpendicular CD, which should measure 10
cm. You may adapt one of the methods from Section 3.6, or use the lines
on the paper to guide you. |
|
6. Through point D, raise perpendicular* EF, which
is parallel* to AB. |
| |
|
|
|
|
|
|
7. Taking point D as zero, measure 10 cm to the left
and 10 cm to the right of point D, along EF. Divide these two distances into
millimetres and mark the main graduations. Once again, the lines
on the paper will help you.
Note: instead of drawing the above lines yourself, you
can use Figure 4. Make an enlarged a copy of
it and cut it out. Glue this figure to the wooden board, with line AB parallel
to the board's longer edge.
|
FIGURE
4

|
8. Make a plumb-line 17 cm long, using very thin thread (such as
a nylon fishing line) and a small weight. Drive in a small nail exactly at point
C on the board, and hang the plumb-line from it. Slightly below the nail, at
K on line CD, drill a hole that a wood-screw will pass through.
9. Make a sighting line* along line AB. To do this, you can drive
thin nails in at points A and B. Or, get two metal strips (you can cut them
from a tin) and cut small, v-shaped notches out of one end of each strip. Then,
bend the other end so that the strips can be attached perpendicular to the board.
Screw them to points A and B, making sure that the v-notches (your sighting
guides) are directly over the two marked points A and B. Align these v-notches
with line AB.
Mark sighting line AB with nails...

|
|
... or with v-notch sighting guides

|
10. Get a wooden staff 2 m long to use as the support, and make a point on
the bottom end. Loosely attach the clinometer board near the top of this staff
with a screw through the hole K you made on line CD in step 8. Tighten the screw
so that the board can be turned around. Check that the head of the screw lies
slightly below the surface of the board so it will not disturb the
plumb-line.
|
|
Attach the board so it can turn easily
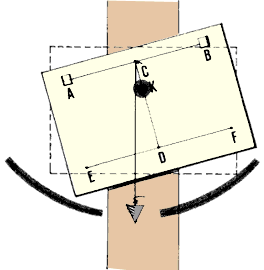
|
|
Make sure the plumb-line swings freely

|
|
11. Clearly mark a reference line* about 25 cm above the pointed
end of the supporting staff, showing the depth to which you need to drive
it into the ground at each station. Measure the distance between this
reference line and the sighting line AB.
12. Then prepare a pole or staff with a reference line and a sighting
line at exactly the same height as line AB. This will be your sighting
pole.
|
|
|
Using the clinometer for measuring a slope in percent
|
|
13. You can measure either uphill or downhill slopes by reading the appropriate
one of the two scales.
14. Place the sighting pole you made in step 12 on point Y of the slope
you are measuring, about 15-20 m away. Drive it in vertically up to the
reference line.
|
|
|
15. At point X, drive your clinometer support vertically into the
ground up to the reference line. With the sighting line, aim at the mark on
the sighting pole. Rotate the board around its screw until you sight the marked
level.
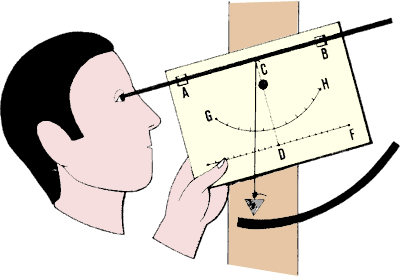
Turn the board until you sight the top of the pole
|
16. Where the plumb-line crosses line EF, read the graduation (in millimetres).
This gives you the slope in percent.
Note: check carefully to see that the plumb-line
hangs freely from its support. The board should rotate without disturbing the
vertical position of the plumb-line.
Using the clinometer to measure a vertical angle in degrees
17. If you must measure a vertical angle in degrees instead of a
slope, you may use the model 4 clinometer (as described above). The only difference
in this case is that you use the curved scale GH (in
Figure 4) rather than the bottom scale.
4.5 How to use the clisimeter
|
|
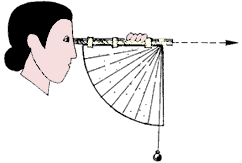
1. The clisimeter is a simple instrument for measuring horizontal
distances, as explained in Section 2.7. It can also be used to measure
a slope or a vertical angle, but it can only give a rough estimate
of these, accurate to within 10 percent.
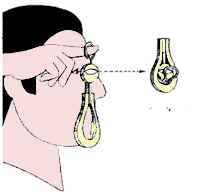
The lyra clisimeter is a commonly used model. It is made up
of a sighting device, an attached ring,
and a weight, shaped like a pear, which keeps the clisimeter
in a vertical position when hung from its ring. The instrument folds neatly
into the weight for transport.
|
|
|
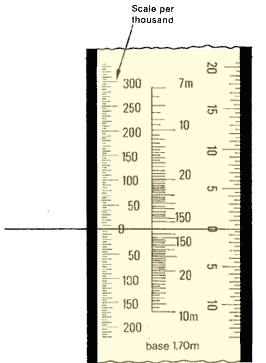
2. When you look through the sighting device, you see three
scales. As described before (see Section 2.7, step 3), the central scale
is used to measure horizontal distances. The other two scales are used to measure
vertical angles and slopes. You will use the left scale, which is
graduated in per thousand (%o) or tenths of percent (%):
The scale inside the clisimeter-use the left scale
to measure slopes
|
|
100 on the scale %o = 10%
or
5% = 50 on the scale %o
Examples
15 per thousand equals 15 � 10 = 1.5
percent
35 per thousand equals 35 � 10 = 3.5 percent
150 per thousand equals 150 � 10 = 15 percent
7 per thousand equals 7 � 10 =
0.7 percent
Note: the right scale is graduated in grades
(G), a unit of measurement which you have not used yet. Full circle is
divided into 400 grades. Up to now we have been using degrees.
There are 360 degrees in a full circle!
|
3. The left scale is graduated from zero in two opposing directions:
- above zero are the positive
graduations for measuring uphill slopes;
- below zero are the negative
graduations for measuring downhill slopes.
Using the clisimeter to measure a slope
You can use the clisimeter by
yourself or with an assistant:
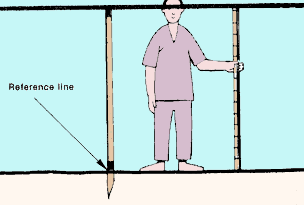
4. If you are working alone, you need a pointed stake clearly marked at two
levels: the reference level above the pointed bottom, showing the depth to which
you will drive the stake into the soil; and the eye level, which
is the vertical measurement from the reference level to your eye
level. It is best to have the eye level at the top of the stake. (This
stake is like the one you learned to make in Section 4.1, step 5.)
Make a sighting pole marked at eye level
|
|
Sight at the marked pole

|
5. If you have an assistant, you can also use a simple rod marked at eye level,
but it will be faster to use your assistant instead of this rod. To do this,
determine the point on your assistant which is at the same level as your own
eyes and sight at that point instead.
Using the clisimeter to lay out a slope
9. You will need an assistant for this method. Sight with the graduation on
the left scale (which corresponds to the slope) at the marked level (on
a rod such as the one described in Section 4.1, step 5, for example) corresponding
to the height of your eyes.
| 10. Ask your assistant to move the marked rod forward or backward
until the eye level line is even with the clisimeter graduation. |
|
11. When the rod is properly aligned, ask your assistant to
mark the point on the ground with a stake. Move up to this stake and repeat
the procedure. |
| |
|
|
Move the sighting pole until you see it
at the
correct graduation 
|
|
|
Note: if you need greater accuracy, you can hang the clisimeter
at fixed height from a stick. If you do this, remember to adjust the marked
level on the rod to this height.
4.6 How to use the optical clinometer
|
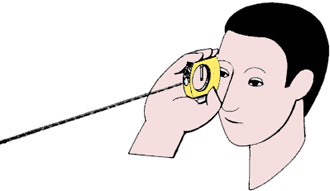
| 1. An optical clinometer is a precise pocket instrument
for measuring vertical angles and estimating tree heights. It is commonly
used by foresters. It can also be used to measure slopes quickly, with a
method similar to that described for the clisimeter (see Section 4.5). |
|
Clinometer
|
| |
|
|
| 2. When you look through the sighting device of the clinometer,
you can see a cross-hair and two scales. The left scale is graduated in
degrees and the right scale is graduated in percent. Both
scales have a positive (+ ) section for measuring uphill slopes and a negative
section (-) for measuring downhill slopes. |
|
Sight through hole
|
| |
|
|
| 3. Keeping both eyes open, sight with one eye through the
optical clinometer, moving it until the cross-hair lines up with the marked
level you wish to measure (such as a rod). With the clinometer
lined up in this position read the graduation at the cross-hair. |
|
Read the graduation at the cross-hair

|
4.7 How to use miscellaneous levelling devices
|
| 1. In Chapter 5, various levelling devices will be discussed.
These devices can also be used to measure a slope. To
set a graded line of slope, see Section 6.9. |
|
Sight with the clinometer
|
| |
|
|
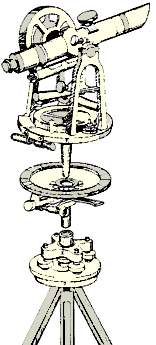
2. In Section 3.5, you learned about
theodolites and how you can use them to measure horizontal angles. Most
theodolites are designed to measure vertical angles as well.
For this purpose, they are fitted with:
- a graduated vertical circle attached to the horizontal
axis of the telescope;
- an extra graduated plate inside this circle for highly
precise measurements.
|
|
Theodolite
|
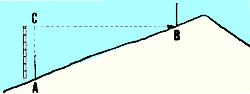
3. Levelling devices help you measure the difference in levels
between two points. After you have measured the horizontal distance
between these points, you can calculate the slope
as explained earlier (see Section 4.0, step 8).
Measuring difference in levels between two points
|
|
|
|
4.8 How to set out and check
verticals
|
| 1. A vertical is a line with a 90� slope. You will often
have to set out verticals, especially when you are building walls for
a canal or building. You have already used vertical lines, to
measure distances over sloping ground for example (see Section 2.6,
step 19). |
|
Most walls are vertical
|
Setting out and checking verticals with a plumb-line
|
| 2. A plumb-line is a simple device which forms
a vertical line*. The idea of the plumb-line is based on the
fact that any heavy object will fall vertically, making a 90�
angle with the horizontal plane at ground level. |
|
3. In a plumb-line, a fairly heavy object, the
plumb, is attached to the end of a thin line.
When the plumb hangs freely without moving, the line is vertical.
|
| |
|
|
Gravity makes objects fall vertically

|
|
A plumb-line
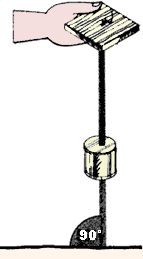
|
Making your own plumb-line
|
|
4. You can make a simple plumb-line from:
- a thin line about 50 cm long, such as a piece of string,
cotton thread, or nylon fishing line; and
- a small but heavy object, such as a stone,
metal nut, or fishing lead.
|
|
5. You can make an improved plumb-line for measuring
buildings in progress and other constructions. Start with a piece of wood
or heavy metal about 10 cm square. |
| |
|
|
A simple plumb-line
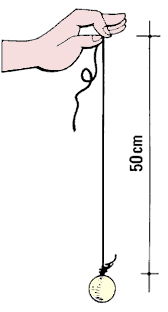
|
|
An improved plumb-line
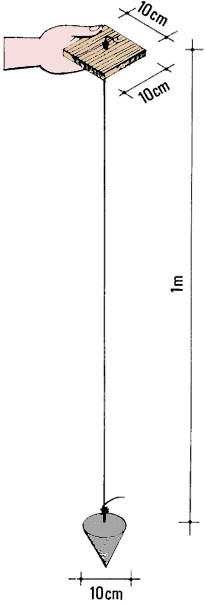
|
| |
|
|
| 6. Find the exact centre of the square piece by drawing
two diagonal lines on it. Drill a small hole through the point where they
cross.
7. To make the plumb, get a heavy, solid block of wood (such as red
acajou) or metal - the largest side of this block should be 10 cm across
or less - if you can, shape the block into a cone.
8. If the block is wooden, drive a small nail into the exact centre
of its top surface. If the block is metal, have a small hook welded
to this point.
9. Attach the end of a thin line (nylon fishing line is strongest)
about 1 m long to this nail or hook on the block and pass the other
end through the central hole of the wooden or metal square piece. Fix
the line on the other side of this hole either by tying it into a heavy
knot or by tying a small piece of wood or metal (such as a nut) on to
its end.
Note: you can change the dimensions of the plumb-line,
depending on the materials you have. The line can be longer, if necessary.
|
|
|
| |
|
|
| |
|
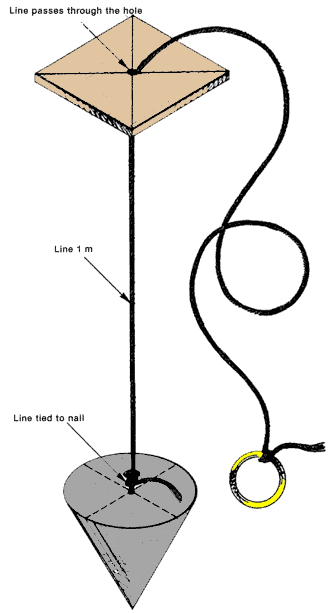 Don't let the end of the line pass through Don't let the end of the line pass through
|
Using a plumb-line to set out a vertical
|
|
10. Remember that a free plumb-line will hang vertically.
11. You can use a simple plumb-line to see if a wall is vertical. To
do this, hold the top end of your plumb-line close to the wall and check
to see if the distance between the wall and the top end of the line is
equal to the distance between the wall and the centre of the weight at
the bottom. This distance will be easier to check if the weight is
pointed on the bottom.
|
|
Checking a vertical with a plumb-line
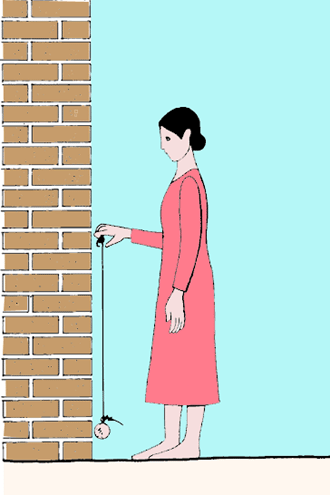
|
| |
|
|
| 12. When using the improved plumb-line along a wall: |
| |
|
|
- if the diameter of the weight is equal to the diameter of the top
square, place one of the sides of the square against the wall. Check
to see that the side of the weight slightly touches the wall;
|
|
- if the diameter of the weight is smaller than the diameter of the
top square, place one of the sides of the square against the wall. Check
to see that the distance from the centre of the weight to the wall equals
half the length of the square's side.
|
| |
|
|
Same-size weight touches the wall
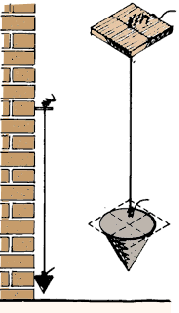
|
|
Measure a smaller weight
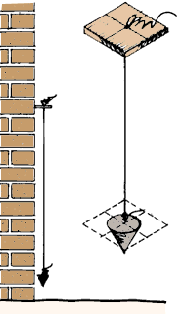
|
Note: if you need to make the plumb-line shorter
to measure along walls of different heights, you can pull the line up through
the centre-hole in the square at the top. Let it back down through the hole
to measure higher walls.
|
Pull up the line to measure shorter verticals
 |
Checking small verticals with a mason's level
13. Some mason's levels (see Section 6.1) have an additional
bubble level for checking verticality. You can use this level
when you are building walls, for example. This method is particularly useful
when the vertical you are checking is fairly small. Hold the mason's level vertically
against the surface you need to check. If the surface is vertical, the bubble
will be at the exact centre of the bubble level.
