7. TOPOGRAPHICAL SURVEYS - PLAN SURVEYING
7.0 Introduction
|
What is a topographical survey?
|
|
|
| 1. A survey of your fish culture site can help you do one
of two things: make a map to help you plan your work; or lay out marks on
the ground that will guide you as you work. |
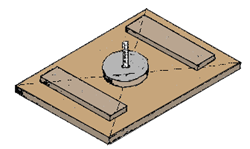
|
Site
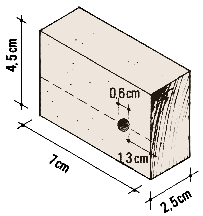
|
| |
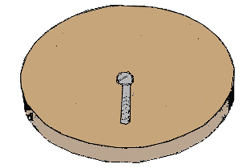
|
|
2. Topographical surveys will help you to make plans or maps
of an area that show:
- the main physical features on the ground, such as rivers, lakes, reservoirs,
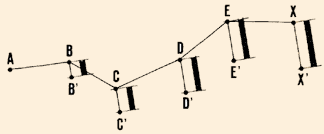
roads, forests or large rocks; or the various features of the fish-farm,
such as ponds, dams, dikes, drainage ditches or sources of water;
- the difference in height between land forms, such as valleys, plains,
hills or slopes; or the difference in height between the features of
the fish-farm. These differences are called the vertical relief.
|
|
Map
|
| |
|
|
| |
|
Vertical profile
|
|
What do topographical surveys involve?
|
|
3. The purpose of the first type of topographical survey is to establish,
on a horizontal plane, the position of one or more points in relation
to the position of one or more other points. To do this, you will measure
horizontal distances and horizontal angles or directions. You will use
a method called plan surveying, which will be explained in this chapter.
|
|
Site
|
| |
|
|
| 4. The purpose of the second type of topographical survey
is to find the elevation (or vertical height) of one or more points above
a definite horizontal plane. To do this, you will measure horizontal distances
and height differences; you may also need to lay out contour lines. You
will use a method called direct levelling, which will be explained in Chapter
8. |
|
Map
|
| |
|
|
| 5. You will learn how to make plans and maps based on the
results of plan surveying and direct levelling in Chapter 9. |
|
Contour map
|
|
Planning your topographical surveys
|
|
6. When you plan a topographical study, the most important rule to remember
is that you must work from the whole to the part, keeping in
mind all of the work you will need to do as you begin the first steps.
Different types of survey require different levels of accuracy, but you
should lay down the first points of each survey as accurately as possible.
You will adjust all the work you do later to agree with these first points.
|
|
Primary points
|
| |
|
|
|
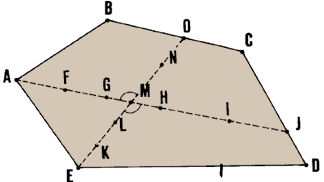
Example
You need to plan survey a fish-farm site.
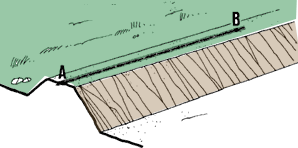
(a) First, you must make a perimeter survey ABCDEA. Besides
these summits and boundaries, add several major points and lines, such
as AJ and EO. They run across the interior to create right angles, which
will help you in your calculations. This survey gives the primary survey
points, which you should determine and plot very accurately.
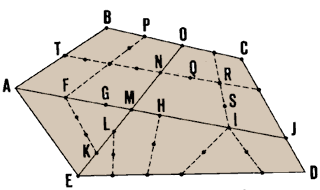
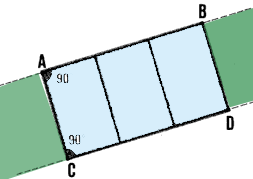
(b) Then, lay out minor lines such as FP and TN. They go between
the major lines to divide the area intoblocks. This gives you the secondary
survey points, which you may determine less accurately.
(c) Finally, survey details in each block using tertiary points,
for which less accuracy is also acceptable.
|
|
Secondary points
|
| |
|
|
| |
|
|
|
7. The way you plan a topographical survey will also depend on its purpose.
You will use a planning method similar to the one described for soil surveys
(see Volume 6, Soil, Section 2.4).
- First make a preliminary or reconnaissance survey. You can use quick
methods without worrying too much about high accuracy.
- Based on the results of this survey you can plan and carry out more
detailed and accurate surveys, such as location surveys and, last of
all, construction surveys.
|
|
|
8. The way you plan a topographical survey will depend on
the subject you need to survey, such as:
- a straight line defined by at least two points, such as the centre-lines
of supply canals, pond dikes, and reservoir dams;
- a series of lines related to each other by horizontal angles and horizontal
distances, such as the centre-lines of pond dikes in a fish-farm;
- an area of land such as a site chosen for the construction of a fish-farm
(also see step 6 above)
|
|
Centre-line of a dike
|
| |
|
|
| |
|
Adjacent pond dikes
|
| |
|
|
| |
|
Pond area
|
| |
|
|
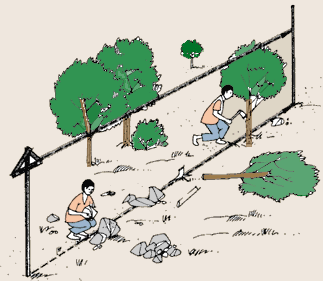
| 9. In open country, you will have no problems in plan surveying
with the methods explained in the next sections. Any of the following methods
should work well. In country with thick forests, however, you will not be
able to use methods for which you need to see several points at the same
time. In such areas, you will also need to rely on existing paths and roads
much more than usual, and you might even need to clear lines of sight through
the vegetation. |
|
Clearing land for a survey
|
|
What are the main methods used in plan surveying?
|
|
10. There are four main methods used in plan surveying. You can fix the
position of a point on the horizontal plane:
- from a single known point, by traversing, a method in which you measure
horizontal distances and azimuths along a zigzag line (see Section 7.1);
- from a single known point, by radiation, a method in which you measure
horizontal distances and azimuths, or horizontal angles (see Section
7.2);
- from a known line, by offset, a method in which you measure horizontal
distances and set out perpendiculars (see Section 7.3);
- from two known points, by triangulation and/or intersection, methods
in which you measure horizontal distances and azimuths, or horizontal
angles (see Section 7.4).
|
|
Open traverse
|
| |
|
|
|
Each of these methods will be explained in the next sections. When you
are choosing a method, you will also need to consider which methods are
suited to the measuring devices you have available. Table
9 will help you select the most suitable plan surveying method, considering
your equipment and abilities, the kind of information you need from your
survey and the type of terrain you are surveying.
|
|
Radiation survey
|
|
TABLE
9
Plan surveying methods
| Section |
Method
|
Basic elements
|
Suitability
|
Remarks
|
| 7.1 |
Traversing, open, closed
|
Traverse sections and stations
|
Flat or wooded terrain
Longitudinal or cross-section profiles
Compass traverse, rapid reconnaissance and details
|
Traverse sections may be of equal lengths,
longer than 25 m and are best at 40 to 100 m
Careful checks for errors needed
|
| 7.2 |
Radiating, central and lateral stations
|
Observation station
|
Small land areas
For location of points only
|
All points should be visible and at angles
greater than 15�
|
| 7.3 |
Offset
|
Chaining line
|
Details surveys next to a chaining line
|
Chaining line should not be more than 35 m
away
|
| 7.4 |
Triangulation
|
Base line
|
Very large land areas
Hilly or open terrains
Inaccessible locations
|
Often combined with traversing and needing
elaborate preliminary reconnaissance
Best with angles of about 60�
|
| 7.5 |
Plane-tabling, traversing, radiating, triangulation
|
|
Reconnaissance and details surveys
Open terrain and good weather
Irregular lines and areas
|
Mapping is done in the field
Rapid method after practice
|
|
7.1 How to survey by traversing
|
What is a traverse?
|
|
1. A traverse line or traverse is a series of straight lines connecting
traverse stations, which are established points along the route of a survey.
A traverse follows a zigzag course, which means it changes direction at
each traverse station.
2. Traversing is a very common surveying method in which traverses are
run for plan surveying. It is particularly suitable to use in flat or
wooded terrain.
|
|
Closed traverse
|
| |
|
|
|
3. There are two kinds of traverses:
- if the traverse forms a closed figure, such as the boundary of a fish-farm
site, it is called a closed traverse;
- if the traverse forms a line with a beginning and an end, such as
the centre-line of a water-supply canal, it is called an open traverse.
|
|
Open traverse
|
Choosing the route of a traverse
|
|
10. When selecting the route a traverse will follow, you should try to:
- make each straight section of the traverse as long as possible (40-100
m);
- make the traverse sections as equal in length as possible;
- avoid very short traverse sections - under 25 m long;
- choose lines which can be measured easily;
- choose lines along routes which avoid obstacles such as heavy vegetation,
rocks, standing crops and property.
|
|
|
| |
|
|
| |
|
|
Surveying an open traverse with a magnetic compass
|
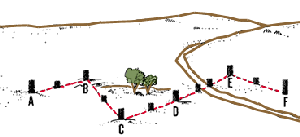
| 11. You need to survey traverse AF for a future water supply
canal. First, walk along the traverse. Mark its course by placing high stakes
about every 50 m. If necessary, place additional stakes at important traverse
stations, such as where the traverse changes direction, where hills or other
changes in elevation reduce visibility between traverse stations, or where
there are particular landscape features such as a road, a river, or rocks.
|
|
Mark the main points
|
| |
|
|
| 12. If necessary, clear any tall vegetation from the path
of the traverse, so that you will be able to see each marked point from
the one before it. |
|
Clear the path and mark details

|
|
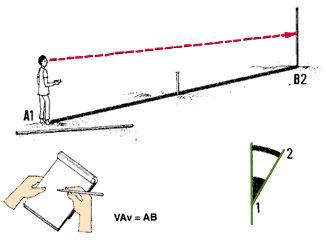
13. Start traversing at the first point A. Remove the ranging pole
and stand at point A. With the magnetic compass, measure the azimuth*
of the line joining point A to point B, the next visible point. Point
A becomes station 1. The direction you measure from there to point B,
or station 2, is called a foresight* (FS) because you are measuring
forward. Note down this value in a table (see step 17).
|
|
FS=AB
|
| |
|
|
|
14. Replace the ranging pole at station 1 (point A) and move to station
2, while measuring the horizontal distance AB by pacing or chaining.
Note this distance down in the table (see step 17).
|
|
Distance AB

|
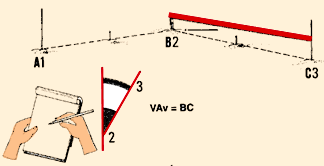
| 15. At station 2 (point B), remove the ranging pole and
stand over the point holding the compass. Look back at station 1 and measure
the azimuth of line BA, which is called a backsight (BS). Then look forward
at the next point C, or station 3, and measure the azimuth of line BC,
a foresight (FS). Measure distance BC while moving forward along the traverse.
Note these values down in the table (see step 17). |
|
BS = BA

|
| |
|
|
| Note: the difference between the foresight and
backsight should be 180�. A difference of only 1 or 2 degrees between
the FS and BS is acceptable and may be corrected later (see step 19).
If the error is greater, you should make the measurement again before
moving on to the next station. |
|
FS = BC
|
| |
|
|
|
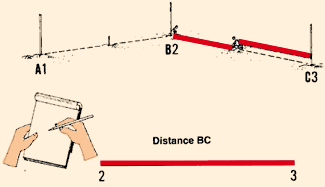
16. Repeat this procedure, measuring horizontal distances from station
to station and measuring two azimuths (a BS and a FS) for each point.
However, from the last station at the end of an open traverse, you will
only have a BS measurement, just as you had only an FS from station
1.
Note: if the land slopes and you need to use a more accurate method,
you can use a special method to measure
or calculate horizontal distances (see
Sections 2.6 and 4.0).
|
|
Distance BC
|
17. You should carefully note down all the measurements you have made in a
field book. You can use a table like the one shown in the example or you can
make a rough sketch of the open traverse on square-ruled millimetric paper,
noting down your measurements next to the correct stations in it.
Example
Measurements observed for the beginning of compass traverse
AX made of 12 stations:
|
Stations
|
Distance (m)
|
Azimuths (degrees)
|
Calculated difference FS/BS (degrees)
|
|
From
|
To
|
Individual
|
Cumulative
|
FS
|
BS
|
|
1
|
2
|
53.6
|
53.6
|
82
|
261
|
179
|
|
2
|
3
|
47.3
|
100.9
|
120
|
301
|
181
|
|
3
|
4
|
65.2
|
166.1
|
66
|
248
|
182
|
|
4
|
5
|
56.8
|
222.9
|
51
|
229
|
178
|
|
5
|
6
|
61.1
|
284.0
|
91
|
270
|
179
|
|
...
|
...
|
...
|
...
|
...
|
...
|
...
|
|
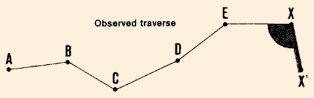
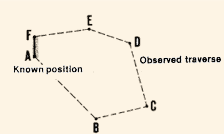
| 18. You must always check on such a compass traverse, particularly
if you do not know the exact position of its starting and ending stations
beforehand from studying previous surveys or existing maps. To check on
your compass traverse, do the following: |
|
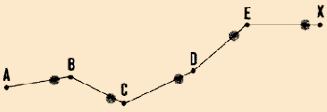
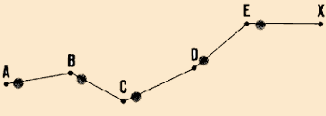
Observed traverse AX
|
| |
|
|
- if the starting and ending traverse stations A and X are unknown,
check on your first traverse by making a second compass traverse in
the opposite direction, from X to A;
|
|
Observed traverse XA
|
| |
|
|
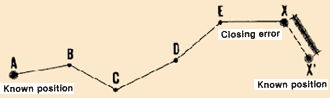
- if these two stations A and X are known, draw the traverse on paper
as you have measured it. To do this, use a protractor for the angles (see Section 3.3)
and an adequate scale for distances (see Section
9.1). Using the known station A, compare the position of the last station
X with its known position X'. If this comparison shows a large error
( the closing error XX'), you will need to adjust the observed traverse
AX. To do this, see the next step.
|
|
Observed traverse AX
|
Adjusting an open traverse
|
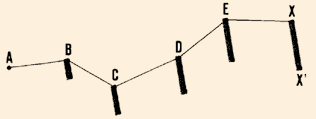
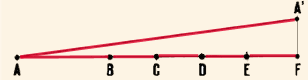
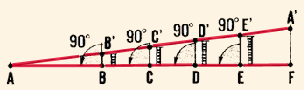
| 19. To adjust the observed traverse AX for the closing error
XX', it is easiest to use the graphic method, as follows: |
|
|
| |
|
|
- on paper, draw a straight horizontal line AX equalling the total measured
length of the observed traverse, drawn at an adequate scale;
|
|
|
| |
|
|
- at X, draw XX' perpendicular to AX and in proportion, in length to
the closing error, using the same scale as above;
|
|
|
| |
|
|
- join A to X' with a straight line;
|
|
|
| |
|
|
- on AX, find lengths AB, BC, CD, DE, and EX in proportion to the field
measurements, using the same scale as above;
|
|
Find the intermediate points BCD and E
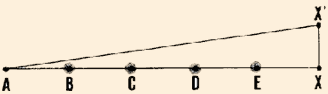
|
| |
|
|
- at points B, C, D, and E, draw lines BB', CC', DD' and EE' perpendicular
to AX;
|
|
Draw perpendiculars BB', CC', DD' and EE' 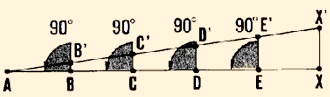
|
| |
|
|
- measure the lengths of lines BB', CC', DD' and EE', which show by
how much you need to adjust each traverse station;
|
|
Measure the perpendiculars
|
- adjust your drawing of the traverse by:
- joining the observed position X of the last traverse station to
its known position X';
|
|
Draw XX'
|
| |
|
|
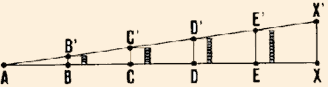
- drawing short lines parallel to XX' through stations
B, C, D and E;
|
|
Draw the other segments parallel to XX'
|
| |
|
|
- marking on these lines the calculated adjustments BB', CC', DD'
and EE', using the same scale as above;
|
|
Measure the distance BB', CC', DD' and EE'
|
| |
|
|
- joining points A, B', C', D', E' and X' to find the adjusted traverse.
|
|
Join the points of the adjusted traverse

|
Surveying a closed traverse with a magnetic compass
|
|
20. You can lay out a closed traverse ABCDEA in exactly the
same way as an open traverse, except that you will connect the last point
to the initial point A.
21. To survey an irregular enclosed area of land ABCDEA (such as a site
for a fish-farm) by compass traversing, proceed as follows:
|
|
|
| |
|
|
- walk over the area and locate traverse stations A, B, C,
D and E;
|
|
|
| |
|
|
- mark them with ranging poles or stakes;
- if necessary, clear away any vegetation so that you can see stations
A and B, B and C, C and D, etc. from each other;
|
|
|
| |
|
|
- remove the ranging pole from point A (station 1) and stand at this
station. Find azimuth AB- a foresight- from the centre of this station
with the compass. Replace the ranging pole exactly at station 1;
|
|
|
- measure distance AB with a measuring line;
|
|
|
| |
|
|
- at point B (station 2), measure azimuth BA - a backsight
and azimuth BC - a foresight;
|
|
|
| |
|
|
- measure distance BC as you move to point C (station 3);
|
|
|
| |
|
|
- proceed in the same way at stations 3, 4 and 5;
- when you reach point A again (station 1), measure azimuth AE - a backsight.
|
|
|
| |
|
|
| Note: during the traverse, you may be able to see
one or more additional stations from the station where you are standing.
If you do, measure the azimuths of the lines running toward them. An example
is line BD from station B. These additional observations are useful checks
on your work. |
|
|
| |
|
|
| |
|
|
22. In a field
book, carefully note down all your measurements. You can use a table similar to the one suggested for the open traverse (see
step 17). You should also make a sketch of the traverse, on a separate square-ruled page, and write in the
measurements. At the same time, check to see that the foresights and backsights differ by
180�.
Example
You have surveyed site ABCDEA with a closed traverse and your field notes
are as follows:
|
Stations
|
Distance (m)
|
Azimuths (degrees)
|
Calculated difference FS/BS (degrees)
|
|
From
|
To
|
FS
|
BS
|
|
1
|
2
|
90.8
|
136
|
315
|
179
|
|
2
|
3
|
53.5
|
78
|
259
|
179
|
|
3
|
4
|
68.7
|
347
|
168
|
179
|
|
4
|
5
|
44.6
|
292
|
110
|
182
|
|
5
|
1
|
63.7
|
241
|
63
|
178
|
23. You have
learned that in any closed polygon*
of N sides, the sum of all the
interior angles should be equal to (N - 2) x 180� (see Section 3.0). This rule will help
you to check your azimuth measurements after you calculate the interior angle for each
station (see Section 3.2, steps 10 and 11).
Example
Using the observations given in the previous example, calculate the sum
of the interior angles of polygon ABCDEA as follows:
|
Station
|
Azimuth differences (degrees)
|
Interior angle (degrees)
|
|
1
|
AB -AE = 136- 63
|
73
|
|
2
|
(BA - BC = 315 - 78 = 237)
|
1231
|
|
3
|
CD - CB = 347 - 259
|
88
|
|
4
|
DE - DC = 292 - 168
|
124
|
|
5
|
EA - ED = 241 - 110
|
131
|
|
|
Sum of interior angles
|
539
|
1 Since the magnetic
north falls inside the angle, you must calculate it as 360� - (the azimuth difference) or
360� - 237� = 123�,
| According to the general rule, the sum of the five interior
angles should be equal to (5-2) x 180� = 3 x 180� = 540�, which closely
agrees with the above result. |
|
Check: Sum of angles = (5 - 2) x 180� = 540�

|
| |
|
|
Adjusting a closed traverse
|
| 24. Starting from station 1 (A), draw the observations of
your compass traverse on square-ruled paper. Use a protractor to measure the azimuths (see Section
3.3), and an adequate scale for the measured
distances (see Section 9.1). If there is a closing error, adjust
your drawing by using the graphic method described for an open traverse
(see step 19, above). |
|
|
| |
|
|
|
Example
For the above example, the closing error is FA. Adjust it as foIlows:
|
|
|
| |
|
|
- using the correct scale, draw a horizontal line AF whose length
equals the total measured length of the observed traverse;
|
|
Draw AF to scale

|
| |
|
|
- at F, draw FA' perpendicular to AF, using the same scale as above.
The length of FA' should be in proportion to the closing error;
|
|
Draw FA' perpendicular to AF

|
| |
|
|
- join A to A' with a straight line;
|
|
Draw AA'

|
| |
|
|
- on AF, draw lengths AB, BC, CD, DE and EF in proportion to the
field measurements, using the same scale as above;
|
|
Find points BCD and E
|
| |
|
|
- at points B, C, D, and E draw lines BB', CC', DD' and EE', which
show how much you must adjust each traverse station;
|
|
Draw and measure the perpendiculars
|
- adjust your drawing of the traverse by:
|
|
|
- joining the observed position F of the last station to its known
position A;
|
|
Draw FA
|
| |
|
|
- drawing short lines parallel to FA through
the other stations B, C, D, and E;
|
|
Draw the other segments parallel to FA
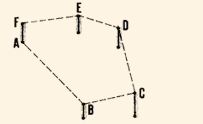
|
| |
|
|
- marking on these lines the calculated adjustments BB', CC', DD'
and EE', using the same scale as above;
|
|
Measure the appropriate lengths
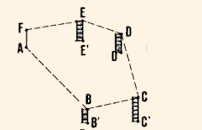
|
| |
|
|
- joining points A, B', C', D', E' and A to determine the adjusted
traverse.
|
|
Join the points of the adjusted traverse

|
7.2 How to survey by radiating
|
What is a radiating survey?
|
| 1. When you plan a survey by radiation, you will choose
one convenient observation station, from which you will be able to see
all the points you need to locate. This method is excellent for surveying
small areas, where you need to locate only points for mapping.
|
|
2. When you make a radiating survey of a polygonal* site,
you connect the observation station to all the summits of this area by
a radiating series of sighting lines. In this way, a number of triangles
are formed. You will measure one horizontal angle and the length of two
sides for each triangle.
|
Choosing the observation station
3. You should be able to reach the observation station easily. This station should also be a
located so that:
- you can see all the summits of
the area you need to survey;
- you can measure the lines joining
it to these summits;
- you can measure the angles formed
by these lines.
4. When choosing the observation station, you should be particularly careful
to avoid any points from which very small radiating angles (less than 15 degrees)
might result.
|
|
|
|
| |
|
|
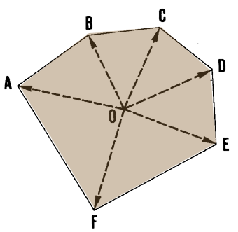
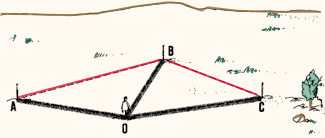
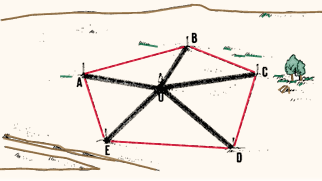
| 5. The observation station 0 can be in a central position,
inside the polygon to be surveyed. In this case, you will measure as many
triangles as there are sides of the polygon. |
|
Number of triangles = number of polygon sides
N = 5
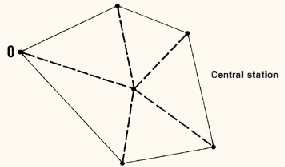
|
| |
|
|
| 6. The observation station 0 can also be in a lateral position
(off to the side). In this case, 0 will be one of the summits of the polygon*.
The number of triangles you need to measure will be the number of sides
to the polygon, minus 2. |
|
Number of triangles = number of sides minus 2
N = 5 - 2 = 3
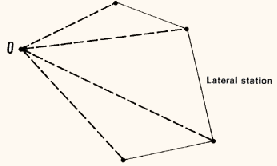
|
|
Carrying out a radiating plan survey with a magnetic compass
|
| 10. Walk over the area you need to survey and choose a convenient
central observation station 0. Clearly mark all summits of the polygon.
Clear any high vegetation along the future radiating lines of sight. |
|
|
| |
|
|
|
11. With your magnetic compass, take a position over the central station
0. Measure the azimuths of the six radiating lines OA, OB, OC, OD, OE
and OF.
12. Measure the horizontal distance over each of these lines.
|
|
|
| |
|
|
|
13. Carefully note down all these measurements in your field-book. You
can use the first three columns of the table given in the example. Then
make a sketch of the area, with the lines and angles and their measurements,
on square-ruled paper.
14. Calculate the value of the angles between successive points
(see 4th column of the table and Section 3.2). Check this by adding all
the values: if you find 360� or a figure close to that, the calculation
is correct.
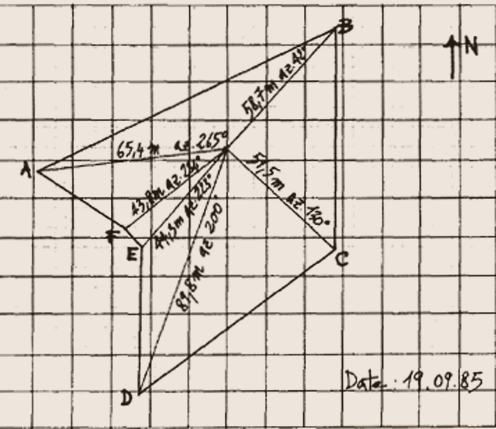
Example
Table for field observations from a radiating survey.
|
Line
|
Distances (m)
|
Azimuths (degrees)
|
Angles (degrees) |
|
From
|
To
|
|
O
|
A
|
65.4
|
265
|
1371
|
|
O
|
B
|
58.7
|
42
|
88
|
|
O
|
C
|
51.5
|
130
|
70
|
|
O
|
D
|
89.8
|
200
|
23
|
|
O
|
E
|
41.3
|
223
|
11
|
|
O
|
F
|
43.8
|
234
|
31
|
|
|
A
|
-
|
265
|
-
|
|
Sum of the interior angles:
|
360
|
1Since magnetic north falls inside angle AOB, it is calculated
as 360� minus the difference of the azimuths.
|
|
|
| |
|
|
|
|
7.3 How to survey by offset
|
What is an offset?
|
| 1. In plan surveying, an offset is a straight line which is
laid out perpendicularly to a line you are chaining. |
|
2. Offsets are mainly used to survey details of the terrain
(such as wells, rocks or trees) which are located close to a chaining line.
Generally, offsets are less than 35 m long. |
| |
|
|
|
|
|
|
Surveying by offset
|
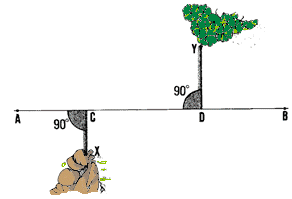
| 3. While chaining line AB, you see two points of interest
on either side of it, X and Y, whose exact positions you want to record.
|
|
|
| |
|
|
| 4. From these points, drop XC and
YD perpendicular to line AB (see Section 3.6). Lines XC and YD are offsets.
|
|
Drop perpendiculars from the points of interest
|
| |
|
|
|
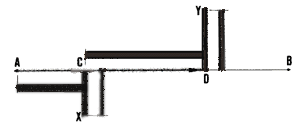
5. Measure horizontal distances AC and CD on line AB. Measure horizontal
distances CX and DY along the offsets.
6. From these measurements you can plot the exact positions of points X and
Y on paper, if line AB is known.
|
|
Measure the distance to plot the points
|
7.4 How to survey by triangulation
|
What is triangulation?
|
| 1. If you use the triangulation method, you will form consecutive
triangles, starting from two known points which you can see from each other.
The straight line joining these two points is called the base line.
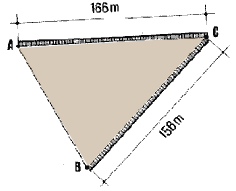
Example
A and B are two points whose positions you know. Therefore, you
can easily survey the baseline AB to find the measurements of the horizontal
distance and magnetic azimuth. AB is 123 m long and azimuth AB = 150�.
|
|
|
| |
|
|
|
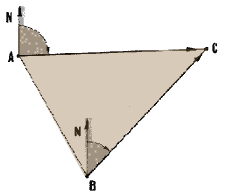
2. To determine the position of a new point C by triangulation, this
new point is joined to the known base line by two new lines, forming a
triangle. You can then find the position of the new point:
- either by measuring the distances of the lines running from the base
line to the point;
- or by measuring the azimuths of the two new straight lines running
from the points A and B to point C.
|
|
Measure distances AC and BC or...
|
| |
|
|
|
Example
It you need to determine the position of C, lay out lines AC and
BC from base line AB. Then you can:
- either measure horizontal distances AC = 166 m and BC = 156 m
to find intersection point C;
- or measure Az AC = 87� and Az BC = 43� to find C at the intersection
point of two lines drawn with these azimuths.
|
|
... measure the azimuths of lines AC and BC
|
| |
|
|
|
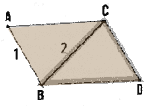
3. To find the positions of other new points, use the same procedure.
As you find the positions of new points, use the most convenient existing
line as the new base line and form new triangles as you work.
|
|
Use BC as the base line for new triangle BCD
|
| |
|
|
|
Example
If you need to determine the position of D, layout triangle BCD
and use BC as the base line. Similarly, to determine points E, F and G,
use base lines CD, DE and EF successively.
|
|
Continue making triangles until you have surveyed
the whole site
|
Using the triangulation method
|
|
4. On terrain with many obstacles such as hills, marshes or high vegetation,
where traversing would be difficult (see Section 7.1), you can use the
triangulation method successfully.
5. When you are traversing, and cannot measure a line directly, you can
use the triangulation method instead.
6. Triangulation makes locating points on opposite sides of a stream
or a lake very easy.
|
|
A good site for a triangulation survey

|
| |
|
|
Using the triangulation method in the field
|
|
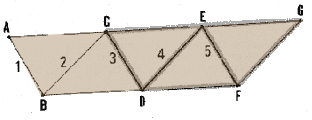
7. The simplest way to use the triangulation method in the field is with
a plane-table(see Section 7.5). You will
learn how to survey by triangulation, using
a plane-table, in Section 9.2.
8. When using the triangulation method, avoid very large angles (over
165�) and very small angles (under 15�). The method works best with angles
of about 60�.
|
|
A plane-table is useful in triangulation
|
| |
7.5 How to use the plane-table
|
What is a plane-table?
|
|
|
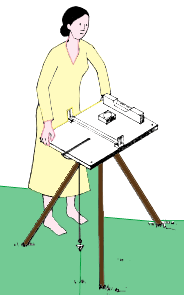
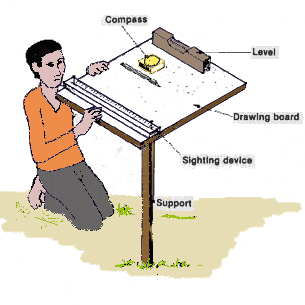
| 1. A plane-table is a horizontal drawing-board mounted on
top of a vertical support. You use it with a sighting device, a spirit
level and a magnetic compass. |
|
Simple plane-table
|
| |
|
Making a very simple plane-table
|
| 2. You can make a very simple plane-table for reconnaissance
surveys from a wooden board and a strong pole. |
|
| |
|
|
|
3. Get a 50 x 60 cm board of soft wood, about 2 cm thick. With sandpaper,
polish one of its surfaces well until it is very smooth. Draw two diagonal
lines lightly across this surface to find the centre of the board.
|
|
|
| |
|
| 4. Get a straight wooden pole about 5 cm in diameter and
1 m long. Shape one end into a point. This will be firmly driven into
the ground at the observation point when you use the plane-table. |
|
| |
|
| 5. Preferably using a brass screw, fix the board, smooth
side up, by its centre-point to the top of the pole. |
|
| |
|
|
| 6. You can make a simple sighting device from an ordinary
ruler about 50 cm long by driving two thin nails vertically into it along
the centre-line for sighting. |
|
Make a sighting device
|
| |
|
|
| 7. You will also need a simple magnetic compass to use with the plane-table. If
you have a spirit level, use it to set up
the top board horizontally. Or simply lay a rounded object such as a small
ball, a glass marble or a pencil on the board's top surface. When the
object remains still, the board is horizontal. |
|
Make sure the board is horizontal

|
Making an improved plane-table
|
|
8. To survey more precisely, you will need a more complicated plane-table
than the one just described. This plane-table will be mounted on a tripod
(a three-legged support) so that:
- you can alter the spread of the tripod's legs to adjust to rough
terrain,
- you can accurately place the drawing board in a horizontal position;
- you can easily orient and rotate the drawing board.
9. You can build a tripod with legs made out of single pieces of wood,
or with adjustable legs. A tripod with adjustable legs is more difficult
to make, but it is better since you can set up the plane-table more
easily on sloping ground by changing the length of the legs.
|
|
An improved plane-table

|
| |
|
|
10. A plane-table with a normal tripod is adequate for surveying
horizontal areas and areas with small slope gradients, which you must
often survey in aquaculture. To make this type of plane-table, you will
need the following materials1:
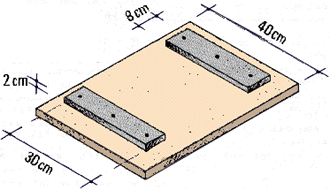
- one board of soft wood, about 40 x 55 cm and 2 cm thick
- three pieces of wood, about 2.5 x 4.5 cm, and
1.4 m long;
- three blocks of wood, about 2.5 x 4.5 cm, and 7 cm long;
- two circular pieces of wood, 15 cm in diameter and 2.5 cm thick;
- several nails or wood screws, both 3.5 to 4 cm long and 6 to 6.5
cm long;
- four bolts, 6 mm in diameter and about 6 cm long;
- four washers and four wing nuts for the bolts.
1Adapted from Using Water Resources, Maryland, USA, VITA
Publications, 1977, pp. 137-140.
|
|
|
| |
|
|
|
11. Get a piece of 40 x 55 cm plywood 2 cm thick to use for the drawing
board. If the plywood you have is thinner than 2 cm, make two battens
(wooden supports) from two pieces of wood 30 x 8 cm and 2 cm thick.
Attach these battens parallel to the 40 cm sides of your board, a few
centimetres in from each side. The wood you use for the board should
be soft enough to allow drawing pins and ordinary pins to go in easily.
You should smooth the top of the board with sandpaper if the surface
is irregular.
|
|
If the board is thin, strengthen it with battens
|
| |
|
|
| |
|
Sand the surface smooth
|
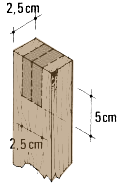
12. Make the three legs from the 1.4 m pieces of wood. Shape
each into a point at one end. On the other end- face of each leg, mark a centre-line
parallel to the 2.5 cm sides. Continue this line 5 cm down either side of the leg. At
these two points, mark a centred perpendicular line 2.5 cm long; connect the end-points of
this 2.5 cm line up the sides of the leg and over the top. Cut out this block you have
marked, which will measure 2.5 x 2.5 x 5 cm, and discard it. Round off the edges of the
two remaining "prongs" of wood which face toward the 2.5 cm side of the leg,
using a knife and sandpaper, for example.
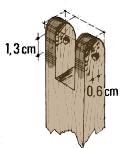
13. On these prongs, drill a 6 mm hole at a point 1.3 cm from the top of the
leg.
|
|
|
Cut out the blocks
|
|
Shape the ends into points

|
| |
|
Round the tops and drill two holes
|
| 14. Make the rotating connection between the drawing board
and the legs with the two circular pieces and the three small blocks of
wood. Drill a 6 mm hole in the centre of one of the 15 cm wooden circles.
Put a 6 mm bolt through the hole making sure the head of the bolt is even
with the top surface of the circle. |
|
Put the bolt through the centre of the disc
|
| |
|
|
| 15. Find the centre of the lower surface of the drawing board
by drawing two diagonals across it from opposite corners. Hold the wooden
circle on this side of the board, with the head of the bolt touching the
centre mark. Nail or screw the wooden circle in place. |
|
Nail the disc to the board so that the bolt sticks
up
|
| |
|
|
|
16. Take the second 15 cm circle and mark the points where you will attach
the legs. To do this, first draw two perpendicular lines across the circle.
They should intersect at the exact centre of the circle. Call them diameters
a and b. With a protractor, using line b as the 0 to 180� line, draw two
more lines from the centre of the circle to the edge at 45� and 135�.
Call them radiuses c and d. They should divide one half of the circle
into four equal, wedge-shaped sections. Then drill a 6 mm hole in the
centre of the circle.
|
|
|
| |
|
| |
|
| |
|
| |
|
17. Drill a 6 mm hole on the centre line of the 4.5 x 7 cm face of each 7 cm
wooden block, 1.3 cm in from one end. Nail or screw these three 7 cm wooden
blocks to the surface of the second wooden circle, so that they join around
the centre-hole in a Y-shape. To do this, align the centre-lines of the blocks'
2.5 x 7 cm faces over the lines a, c and d that you drew in step 16. The ends
with the holes should be towards the edge of the circle.
Drill a hole in each block
|
|
Attach the blocks to the disc, following
the lines you have drawn
|
| |
|
|
|
18. Place this wooden circle, with the blocks facing you, against the
circle already fixed to the underside of the board. Pass the bolt in the
first circle through the centre-hole of the second circle. Add a washer
and a wing nut to it and tighten them securely.
|
|
Mount the disc on the board
|
| |
|
|
|
19. Align the holes in the three legs with the holes in the three blocks
of wood on the underside of the board, and attach the legs with bolts,
washers and wing nuts to the blocks. Your plane-table is now ready to
use.
20. You will also need a small spirit level, a magnetic compass, a sighting
device called an alidade. You have already learned a one kind of alidade
(see Section 3.1), but this one will be slightly different.
|
|
|
|
Making your own alidade
|
| 21. With the plane-table described above, you should use an
alidade about 40 cm long. Get a straight strip of wood 40 cm long, 5 cm
wide and 0.5 to 1 cm thick. Find the centre-line, then measure 5 cm from
each end and draw a line from the edge of the alidade to the centre-line.
Cut out the section you have marked off. |
|
|
| |
|
|
|
22. Get a clean, empty metal tin and remove its top and bottom. Cut this
tube vertically and flatten it out to make a sheet of metal.
23. From this sheet, cut out two pieces 5 cm x 12 cm each. Mark the centre-line
lightly on each, using a nail to scratch the line.
|
|
|
| |
|
|
| |
|
|
| |
|
|
| 24. On one of these pieces, cut an 8 cm slit along the center
line, starting about 1 cm in from the 5 cm edge. |
|
25. On the second piece, cut out a 3 cm x 8 cm window, as
shown in the drawing. |
| |
|
|
|
|
|
|
| |
|
|
| 26 . On the piece with the window, make a small hole at each
end of the window "frame", along the centre-line. Thread a thin
line (such as wire or nylon fishing line) through these two holes and knot
the ends at the back. This line should now exactly follow the centre-line
of the window. |
|
|
|
27. On each metal piece, use a nail to draw a fairly deep line perpendicular
to the centre-line, at a point 2 cm from the end without a slit or window.
Then make three small holes parallel to this line and between the line
and the end of the piece, using a hammer and nail. Sharply bend this end
of the metal along the deep line, until it forms a right angle with the
rest of the piece.
|
|
|
| |
|
|
|
28. Attach the metal pieces to the ends of the wooden strip you prepared
in step 21. Hold them in place with a small screw in each of the holes you
have made in the metal. Make sure that:
- the vertical sides of the metal pieces are at right angles to the
straight edge; and
- the centre-lines of each end piece ( marked by the slit and the wire)
line up with the centre-line of the wooden strip.
You will use the alidade set flat on the plane-table. You will sight
through the slit at the wire. You will draw the line along the centre-line
of the wooden strip.
|
|
|
| |
|
|
Using the plane-table
|
29. You can use the plane-table in two different ways, depending
on the type of survey you are making:
- in reconnaissance surveys, to make maps and plans quickly in the field;
- in later surveys, to fill in details after you have determined the
primary points.
The plane-table can also be used for measuring
horizontal angles.
30. Before you plan survey with the plane-table, you will need to:
- fix a piece of drawing paper on the top of the board;
- set the plane-table up over the station point;
- level the drawing board, or make it horizontal;
- orient the drawing board to face the line you want to survey.
You will learn more about each of these procedures later (see steps 34-47).
|
|
|
|
31. When you are ready to start surveying with your plane-table, you
will then:
- sight with the alidade at a point you have chosen (a foresight);
|
|
|
| |
|
|
- draw this line of sight on the drawing board with a well-sharpened
pencil that has a hard lead;
- measure the horizontal distance from the station to the point;
- transfer this distance to the line you have drawn, using an appropriate
scale;
- if necessary, move to another station, and take a backsight along
the line you have drawn;
- repeat the above procedure for all the lines you need to survey,.
You will learn more about each of these procedures later (see
Chapter 9).
|
|
|
| |
|
|
|
What are the advantages of plane-tabling?
|
|
32. Compared with other methods of plan surveying, plane-tabling is
better in some ways because:
- it is the only method with which you can make a plan or map in the
field;
- you need to find fewer points, as you draw the map while you survey;
- you can plot irregular lines and areas fairly easily and accurately;
- you can work quickly, once you learn how to use the method;
- you do not have to measure angles, so that you avoid several possible
sources of error;
- you plot everything in the field, and so avoid missing any features
you need to measure;
- you can easily check on the location of points you have plotted.
|
|
|
What are the disadvantages of plane-tabling?
|
33. Several disadvantages to plane-tabling are that:
- the plane-table and its extra equipment are heavy and fairly awkward
to carry;
- learning how to use the plane-table correctly takes some time;
- you can only use the method in fairly open country, where you can
see most of the points you are surveying;
- you cannot use the method in bad weather conditions, such as heavy
rains or high winds.
|
|
|
| |
|
|
Covering the board with drawing paper
|
|
34. You should try to find the best quality drawing paper possible to
use with the plane-table. Since the paper will be exposed to outdoor conditions,
you should prepare it to make it more resistant to changes in the humidity
of the air. With a wet cloth, lightly dampen the paper and dry it several
times before you use it. This is called seasoning the paper.
Note: be careful not to make the paper too wet when you season
it.
35. Cut the sheet of drawing paper to a size 20 cm larger than the dimensions
of your drawing board.
36. Cut the four corners of the paper off diagonally. To do this, measure
20 cm from each corner along its two sides, and mark the points. Join
these points by diagonal lines, and cut along these lines.
|
|
|
| |
|
|
|
37. For the last time, slightly dampen the back of the drawing sheet,
then place it over the board. Stretch it well (taking care not to tear
it) and secure the edges under the board with drawing pins. This will
keep the paper from moving and prevent the wind from getting underneath
it.
|
|
|
| |
|
|
|
38. If you plan to work in the field for several days with the same piece
of drawing paper, you should protect it by covering it with a sheet of
smooth, heavy paper. As you work in the field, you can tear off pieces
of this cover sheet to expose the drawing paper as you need it.
39. You should keep the plane-table in a waterproof canvas bag when you
carry it in the field.
|
|
|
Setting up the plane-table
|
|
40. If you decide to start the survey from a selected station, first
set up the plane-table over this station.
Note: you may need to set up the plane-table so that a point
drawn on it is exactly over a corresponding ground point. You can use
a V-shaped metal arm and a plumb-line, which you can easily make yourself.
|
|
|
| |
|
|
|
Otherwise, you can use calipers and a plumb-line. The metal arm or calipers
should be placed with one tip touching the point on the plane-table and
the other tip on the underside of the table. Hang the plumb-line from
the point indicated on the underside of the table, and move the table
until the plumb-line is directly over the ground point.
41. Spread the tripod legs well apart, and plant them firmly in the ground.
The drawing board should be waist-high, so that you may bend over it without
resting against it.
|
|
|
| |
|
|
| 42. Rotate the table top so that the paper is in a position
that allows you to draw the whole area you need to survey on it. |
|
|
| |
|
|
|
43. Choose the scale you will use (see Section
9.1), making sure it will allow you to plot even the most distant
point on the paper. You can first walk quickly over the terrain you will
survey to check the distances by pacing
so you can decide on the right scale to use (see Section 2.2).
|
|
 Level
the table-top in both directions
Level
the table-top in both directions
|
| |
|
|
| 44. Level the board with the spirit level, making
it as horizontal as possible. To do this, first place the spirit level along
one side of the board, parallel to two legs of the tripod and adjust the
table to a horizontal position. Then place the level along the side perpendicular
to that pointing toward the third leg of the tripod and adjust again. Repeat
this process until the board is horizontal. |
|
|
Orienting the plane-table
|
| 45. You can orient the plane-table either
by using a magnetic compass or by backsighting. Usually, the board is first
oriented roughly by compass, and then more precisely by backsighting. |
|
|
| |
|
|
|
46. If you use a magnetic compass (see
Section 3.2), rotate the compass until the direction of the needle lines
up with the direction of south-north, or the 180� to 360� direction. Draw
a line on the drawing paper showing this direction. Draw another line
in the same direction on another part of the paper. Mark the north direction
on these lines with an arrow and the letter N.
Note: remember to keep away from any materials
which could have an effect on the magnetic needle of the compass (see
Section 3.2, step 17).
|
|
|
| |
|
|
| 47. If at a surveying station you know
the direction of a line which you have already plotted on the board, you
can use that line to orient the plane-table by taking a backsight. It is
the most precise way of orienting the plane- table and you should use it
whenever possible. |
|
|
| |
|
|
|
Example
From station A, you have already plotted line ab. Set up the plane-table
at station B. Place the centre-line of the alidade along line ba on the
board. Rotate the board until the line of sight on the alidade lines up
with line BA on the ground. The table is now oriented. You can proceed
to survey and plot new points.
|
|
Station B

|
Plane-tabling methods for reconnaissance surveys
48. During reconnaissance surveys, you can use plane-tabling to quickly map
out areas and open traverses. The survey will proceed by one of the methods
described earlier in this chapter or a combination of them. This method may
be:
Plane-tabling for plotting details
|
| 49. When you have finished the reconnaissance survey and accurately
mapped the main stations, you can further use plane-tabling to locate details
such as rocks, buildings, a well or a group of trees. |
|
|
| |
|
|
|
50. To do this, set up the plane-table at each of the main stations in
turn, and draw sighting lines to each of these features.
51. You can locate each detail on the drawing board by finding the intersection
point of at least three sighting lines. You will not have to take any
more measurements.
|
|
ABCD main stations

|
Example
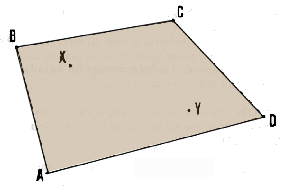
During a reconnaissance survey you have accurately mapped the fish-farm
site ABCDA using your plane-table. You want to add the exact positions of a
rock outcrop X and a group of buildings Y. Proceed as follows:
- set up the plane-table over point A, orienting it by backsighting known
lines AB and AD;
- draw lines AX and AY;
- move the plane-table to point B, orienting by lines BA and BC and draw
line BX;
- move to point C and draw lines CX and CY;
- move to point D and draw line DY;
- determine the position of X at the intersection of AX, BX and CX;
- determine the position of Y at the intersection of CY, DY and AY.
Site ABCDA
|
|
Sight from point A
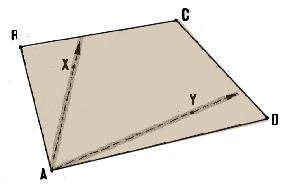
|
| |
|
|
Sight from point B

|
|
Sight from point C

|
| |
|
|
Sight from point D

|
|
The intersections determine points X and Y
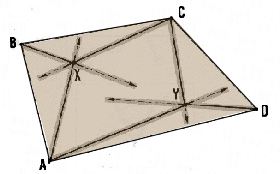
|
|
Measuring horizontal angles by plane-tabling
|
|
52. You can measure horizontal angles fairly accurately by drawing sighting
lines on a plane-table and measuring this angle with a protractor (see Section 3.3).
|
|
Draw ab
|
| |
|
|
|
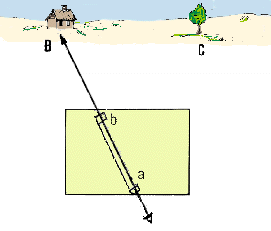
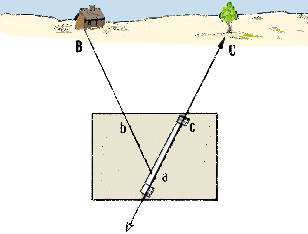
Example
- You need to measure angle BAC formed by straight lines
AB and AC, which have been well-marked in the field. Begin by setting
up the plane-table at station A.
- Place the alidade so that it passes through point a, and sight
at point B, and draw line ab.
|
|
Draw ac
|
| |
|
|
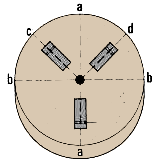
- With the alidade passing through point a, sight at point C and
draw line ac.
- Measure angle bac with a protractor.
|
|
Measure bac

|
